Advertisements
Advertisements
Question
In Figure 1, AP, AQ and BC are tangents to the circle. If AB = 5 cm, AC = 6 cm and BC
= 4 cm, then the length of AP (in cm) is

Options
A. 7.5
B. 15
C. 10
D. 9
Solution

AP, AQ and BC are tangents to the circle. Suppose BC touch the circle at R.
It is given that AB = 5 cm, AC = 6 cm and BC = 4 cm.
We know that, the lengths of tangents drawn from an external point to a circle are equal.
∴ AP = AQ … (1)
PB = BR … (2)
CQ = CR .... (3)
2AP = AP + AP
∴ 2AP = AP + AQ [Using (1)]
⇒ 2AP = (AB + PB) + (AC + CQ)
⇒ 2AP = (AB + BR) + (AC + CR) [Using (2) and (3)]
⇒ 2AP = AB + (BR + CR) + AC
⇒ 2AP = AB + BC + AC
⇒ 2AP = 5 cm + 4 cm + 6 cm
⇒ 2AP = 15 cm
⇒ AP = 7.5 cm
Thus, the length of AP is 7.5 cm.
Hence, the correct answer is A.
APPEARS IN
RELATED QUESTIONS
The common point of a tangent to a circle and the circle is called ______.
In the given figure O is the centre of the circle and AB is a tangent at B. If AB = 15 cm and AC = 7.5 cm. Calculate the radius of a circle.

The given figure shows a circle with centre O and BCD is tangent to it at C. Show that : ∠ACD + ∠BAC = 90°.

What is the distance between two parallel tangents of a circle having radius 4.5 cm ? Justify your answer.
In the given Figure, AB and AC are tangents to the circle with centre O such that ∠BAC = 40°. Then ∠BOC is equal to ______

In the given figure, diameter AB and chord CD of a circle meet at P. PT is a tangent to the circle at T. CD = 7.8 cm, PD = 5 cm, PB = 4 cm. Find the length of tangent PT.

In the figure, PQ and PR are tangents to a circle with centre A. If ∠QPA=27°, then ∠QAR equals to ______


In the above figure, seg AB and seg AD are tangent segments drawn to a circle with centre C from exterior point A, then prove that: ∠A = `1/2` [m(arc BYD) - m(arc BXD)]
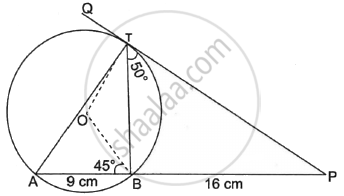
In the given figure, O is the centre of the circle. PQ is a tangent to the circle at T. Chord AB produced meets the tangent at P.
AB = 9 cm, BP = 16 cm, ∠PTB = 50° ∠OBA = 45°
Find:
- Length of PT
- ∠BAT
- ∠BOT
- ∠ABT
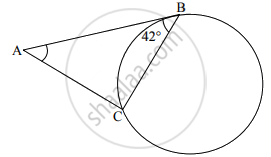
In the given figure, AB and AC are tangents to the circle. If ∠ABC = 42°, then the measure of ∠BAC is ______.
