Advertisements
Advertisements
Question
In figure ABC and DBC are two triangles on the same base BC. Prove that
`"Area (ΔABC)"/"Area (ΔDBC)" = "AO"/"DO"`.
Solution
In ΔAOL and ΔDOM
∠ALO = ∠DMO, ...(90° each)
∠AOL = ∠DOM,
(Vertically opposite 2 sides)
(Vert. opp-angles)
∴ ΔAOL ∼ ΔDOM
∴ `"AL"/"DM" = "AO"/"DO"` ...(1)
If two Δ's are similar the ratio between their corresponding sides is the same.
Now, `"area (ΔABC)"/"area (ΔDBC)" = ((1)/(2) xx "BC" xx "AL")/((1)/(2) xx "BC" xx "Dm") = "AL"/"DM"`
From (1), we get
`"area (ΔABC)"/"area (ΔDBC)" = "AO"/"DO"`.
Hence proved.
APPEARS IN
RELATED QUESTIONS
In quadrilateral ABCD, the diagonals AC and BD intersect each other at point O. If AO = 2CO and BO = 2DO; show that: OA × OD = OB × OC.
Angle BAC of triangle ABC is obtuse and AB = AC. P is a point in BC such that PC = 12 cm. PQ and PR are perpendiculars to sides AB and AC respectively. If PQ = 15 cm and PR = 9 cm; find the length of PB.
In the following figure, XY is parallel to BC, AX = 9 cm, XB = 4.5 cm and BC = 18 cm.

Find :
- `(AY)/(YC)`
- `(YC)/(AC)`
- XY
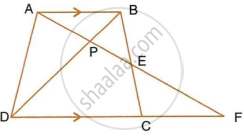
In the following figure, ABCD to a trapezium with AB || DC. If AB = 9 cm, DC = 18 cm, CF = 13.5 cm, AP = 6 cm and BE = 15 cm.
Calculate:
- EC
- AF
- PE
Triangle ABC is similar to triangle PQR. If AD and PM are altitudes of the two triangles, prove that : `(AB)/(PQ) = (AD)/(PM)`.
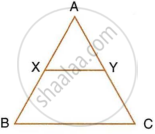
In the following figure, ∠AXY = ∠AYX. If `(BX)/(AX) = (CY)/(AY)`, show that triangle ABC is isosceles.
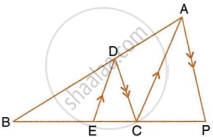
In the following figure, DE || AC and DC || AP. Prove that : `(BE)/(EC) = (BC)/(CP)`.
In the given figure, AB and DE are perpendiculars to BC.
If AB = 6 cm, DE = 4 cm and AC = 15 cm. Calculate CD.

Triangles ABC and DEF are similar.
If area (ΔABC) = 36 cm2, area (ΔDEF) = 64 cm2 and DE = 6.2 cm, find AB.
In the given figure ΔABC and ΔAMP are right angled at B and M respectively.
Given AC = 10 cm, AP = 15 cm and PM = 12 cm.
(i) Prove ΔABC ∼ Δ AMP.
(ii) Find AB and BC.
