Advertisements
Advertisements
Question
In figure, O is the centre of a circle of radius 5 cm, T is a point such that OT = 13 cm and OT intersects the circle at E. If AB is the tangent to the circle at E, find the length of AB.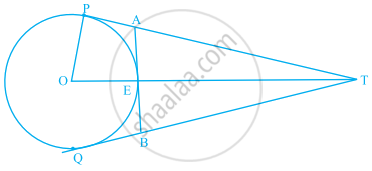
Solution
Given: A circle with center O and radius = 5 cm T is a point, OT = 13 cm. OT intersects the circle at E and AB is the tangent to the circle at E.
To Find: Length of AB
OP ⊥ PT ...[Tangent at a point on the circle is perpendicular to the radius through point of contact]
By pythagoras theorem in ∆OPT right angled at P
(OT)2 = (OP)2 + (PT)2
(13)2 = (5)2 + (PT)2
(PT)2 = 169 – 25 = 144
PT = 12 cm
PT = TQ = 12 cm ...[Tangents drawn from an external point to a circle are equal]
Now, OT = OE + ET
ET = OT – OE
= 13 – 5
= 8 cm
Now, as Tangents drawn from an external point to a circle are equal.
AE = PA ...[1]
EB = BQ ...[2]
Also OE ⊥ AB ...[Tangent at a point on the circle is perpendicular to the radius through point of contact]
∠AEO = 90°
∠AEO + ∠AET = 180° ...[By linear Pair]
∠AET = 90°
In ΔAET By Pythagoras Theorem
(AT)2 = (AE)2 + (ET)2 ...[Here AE = PA as tangents drawn from an external point to a circle are equal]
(PT – PA)2 = (PA)2 + (ET)2
(12 – PA)2 = (PA)2 + (8)2 ...[From 1]
144 + (PA)2 – 24PA = (PA)2 + 64
24PA = 80 ...[3]
∠AET + ∠BET = 180° ...[Linear Pair]
90° + ∠BET = 180°
∠BET = 90°
In ΔBET, By Pythagoras Theorem
(BT)2 = (BE)2 + (ET)2
(TQ – BQ)2 = (BQ)2 + (ET)2 ...[From 2]
(12 – BQ)2 = (BQ)2 + (8)2
144 + (BQ)2 – 24BQ = (BQ)2 + 64
24BQ = 80 ...[4]
So, AB = AE + BE
AB = PA + BQ ...[From 1 and 2]
AB = `10/3 + 10/3` ...[From 3 and 4]
AB = `20/3` cm
APPEARS IN
RELATED QUESTIONS
Draw a circle and two lines parallel to a given line such that one is a tangent and the other, a secant to the circle.
In the given figure, find TP if AT = 16 cm and AB = 12 cm.

In fig. 5 is a chord AB of a circle, with centre O and radius 10 cm, that subtends a right angle at the centre of the circle. Find the area of the minor segment AQBP. Hence find the area of major segment ALBQA. (use π = 3.14)

Prove that the tangent drawn at the mid-point of an arc of a circle is parallel to the chord joining the end points of the arc.
If Δ ABC is isosceles with AB = AC and C (O, r) is the incircle of the ΔABC touching BC at L,prove that L bisects BC.
In the given figure, BDC is a tangent to the given circle at point D such that BD = 30 cm and CD = 7 cm. The other tangents BE and CF are drawn respectively from B and C to the circle and meet when produced at A making BAC a right angle triangle. Calculate (ii) radius of the circle.

Two chords AB and CD of lengths 6cm and 12cm are drawn parallel inside the circle. If the distance between the chords of the circle is 3cm, find the radius of the circle.
In following fig., PT is a tangent to the circle at T and PAB is a secant to the same circle. If PA = 4cm and AB = Scm, find PT.
Find the value of ∠DCE.

In the given figure, PT is a tangent to the circle at T, chord BA is produced to meet the tangent at P. Perpendicular BC bisects the chord TA at C. If PA = 9 cm and TB = 7 cm, find the lengths of:
- AB
- PT

