Advertisements
Advertisements
Questions
In figure, the common tangent, AB and CD to two circles with centres O and O' intersect at E. Prove that the points O, E, O' are collinear.
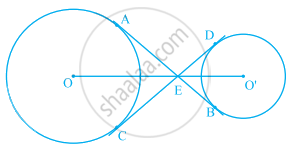
The common tangents AB and CD to two circles with centres O and O' intersect at E between their centres. Prove that the points O, E and O' are collinear.
Solution
Join AO, OC and O’D, O’B.
Now, in ∆EO’D and ∆EO’B,
O’D = O’B
O’E = O’E
ED = EB ...[Tangents drawn from an external point to the circle are equal in length]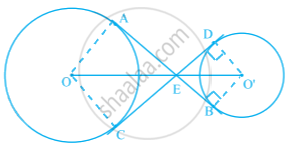
∴ EO’D ≅ ∆ EO’B ...[By SSS congruence criterion]
⇒ ∠O’ED = ∠O’EB ...(i)
i.e., O’E is the angle bisector of ∠DEB.
Similarly, OE is the angle bisector of ∠AEC.
Now, in quadrilateral DEBO’.
∠O’DE = ∠O’BE = 90° ...[CED is a tangent to the circle and O’D is the radius, i.e., O’D ⊥ CED]
⇒ ∠O’DE + ∠O’BE = 180°
∴ ∠DEB + ∠DO’B = 180° ...(ii) [∵ DEBO’ is cyclic quadrilateral]
Since, AB is a straight line.
∴ ∠AED + ∠DEB = 180°
⇒ ∠AED + 180° – ∠DO’B = 180° ...[From (ii)]
⇒ ∠AED = ∠DO’B ...(iii)
Similarly, ∠AED = ∠AOC ...(iv)
Again from equation (ii),
∠DEB = 180° – ∠DO’B
Dividing by 2 on both sides, we get
`1/2 ∠"DEB" = 90^circ - 1/2 ∠"DO'B"`
⇒ ∠DE'O = 90° `-1/2` ∠DO'B ...(v) [∵ O'E is the angle bisector of ∠DEB i.e. `1/2` ∠DEB = ∠DEO']
Similarly, ∠AEC = 180° – ∠AOC
Dividing by 2 on both sides, we get
`1/2 ∠"AEC" = 90^circ - 1/2 ∠"AOC"`
⇒ `∠"AEO" = 90^circ - 1/2 ∠"AOC"` ...(vi) [∵ OE is the angle bisector of ∠AEC i.e., `1/2 ∠"AEC" = ∠"AEO"`]
Now, ∠AED + ∠DEO' + ∠AEO = ∠AED + `(90^circ - 1/2 ∠"DO'B") + (90^circ - 1/2 ∠"AOC")`
= `∠"AED" + 180^circ - 1/2 (∠"DO'B" + ∠"AOC")`
= `∠"AED" + 180^circ - 1/2 (∠"AED" + ∠"AED")` ...[From equation (iii) and (iv)]
= `∠"AED" + 180^circ - 1/2 (2 xx ∠"AED")`
= ∠AED + 180° – ∠AED = 180°
∴ ∠AED + ∠DEO' + ∠AEO = 180°
So, OEO’ is straight line.
Hence, O, E and O’ are collinear.
APPEARS IN
RELATED QUESTIONS
From a point Q, 13 cm away from the centre of a circle, the length of tangent PQ to the circle is 12 cm. The radius of the circle (in cm) is
In Figure 1, AP, AQ and BC are tangents to the circle. If AB = 5 cm, AC = 6 cm and BC
= 4 cm, then the length of AP (in cm) is

In the given Figure, AB and AC are tangents to the circle with centre O such that ∠BAC = 40°. Then ∠BOC is equal to ______

How many common tangents can be drawn to two circles, touching each
other externally?
Two chords AB and CD of lengths 6cm and 12cm are drawn parallel inside the circle. If the distance between the chords of the circle is 3cm, find the radius of the circle.
In fig., PT is a tangent to the circle at T and PAB is a secant to the same circle. If PB = 9cm and AB = 5 cm, find PT.
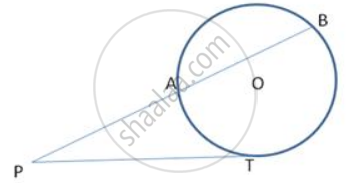
In followinf fig., two concentric circles with centre 0 are of radii 5 cm and 3 cm. from an external point P, tangents PA and PB are drawn to these circles. If AP = 12cm, find BP.

ABC is a right triangle with angle B = 90º. A circle with BC as diameter meets by hypotenuse AC at point D. Prove that: BD2 = AD × DC.
In the following figure, seg AB is a diameter of the circle, m (arc AKC) = 40°. Find the value of m (arc BMC).

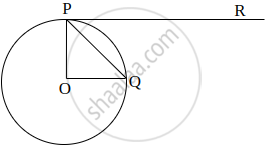
If O is centre of a circle and Chord PQ makes an angle 50° with the tangent PR at the point of contact P, then the angle subtended by the chord at the centre is ______.