Advertisements
Advertisements
Question
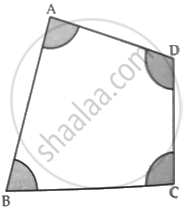
In the following figure, if m(arc DXE) = 120° and m(arc AYC) = 60°. Find ∠DBE.

Solution

By inscribed angle theorem,
m∠AEB=(1/2) × m∠AYC=(1/2) × 60o = 30o ...(1)
m∠EAD=(1/2) × m∠DXE=(1/2) × 120o = 60o ...(2)
∠DBE + ∠AEB = ∠EAD ....(by Exterior Angle Theorem)
⇒ m∠DBE + 30o = 60o
⇒ m∠DBE = 60o - 30o = 30o
APPEARS IN
RELATED QUESTIONS
Find the area of a sector of a circle with radius 6 cm if angle of the sector is 60° [Use `pi = 22/7`]
The length of the minute hand of a clock is 14 cm. Find the area swept by the minute hand in 5 minutes. [Use `pi = 22/7`]
A chord of a circle of radius 10 cm subtends a right angle at the centre. Find the area of the corresponding:
Major sector [Use π = 3.14]
To warn ships for underwater rocks, a lighthouse spreads a red coloured light over a sector of angle 80° to a distance of 16.5 km. Find the area of the sea over which the ships warned. [Use π = 3.14]
A round table cover has six equal designs, as shown in figure. If the radius of the cover is 28 cm, find the cost of making the designs at the rate of Rs.0.35 per cm2. [Use `sqrt3 = 1.7`]
A sector of circle of radius 4cm contains an angle of 30°. Find the area of sector
A sector of 56° cut out from a circle contains area of 4.4 cm2. Find the radius of the circle
AB is a chord of a circle with centre O and radius 4 cm. AB is of length 4 cm and divides the circle into two segments. Find the area of the minor segment.

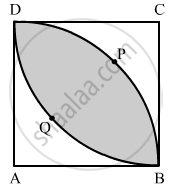
In the given figure, ABCD is a square of side 7 cm, DPBA and DQBC are quadrants of circles each of the radius 7 cm. Find the area of shaded region.
The area of the sector of a circle of radius 10.5 cm is 69.3 cm2. Find the central angle of the sector.
The areas of two sectors of two different circles are equal. Is it necessary that their corresponding arc lengths are equal? Why?
Find the area of the sector of a circle of radius 5 cm, if the corresponding arc length is 3.5 cm.
The central angles of two sectors of circles of radii 7 cm and 21 cm are respectively 120° and 40°. Find the areas of the two sectors as well as the lengths of the corresponding arcs. What do you observe?
Find the perimeter of a quadrant of a circle of radius 14 cm.
The number of revolutions made by a circular wheel of radius 0.25 m in rolling a distance of 11 km is ______.
In the given figure, arcs have been drawn of radius 7 cm each with vertices A, B, C and D of quadrilateral ABCD as centres. Find the area of the shaded region.