Advertisements
Advertisements
Question
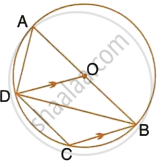
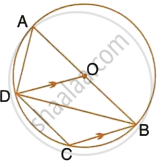
In the given figure, AB is a diameter of the circle with centre O. DO is parallel to CB and ∠DCB = 120°.
Calculate : ∠ADC
Also, show that the ΔAOD is an equilateral triangle.
Solution
∠ABD + ∠DBC = 30° + 30° = 60°
`=>` ∠ABC = 60°
In cyclic quadrilateral ABCD,
∠ADC + ∠ABC = 180°
(Pair of opposite angles in a cyclic quadrilateral are supplementary)
`=>` ∠ADC = 180° – 60° = 120°
In ∆AOD, OA = OD (Radii of the same circle)
∠AOD = ∠DAO Or ∠DAB = 60° [Proved in (i)]
∠AOD = 60°
`=>` ∠ADO = ∠AOD = ∠DAO = 60°
∴ ∆AOD is an equilateral triangle.
APPEARS IN
RELATED QUESTIONS
Calculate the area of the shaded region, if the diameter of the semicircle is equal to 14 cm. Take `pi = 22/7`

Prove that the rhombus, inscribed in a circle, is a square.
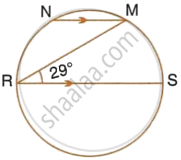
In the given figure, RS is a diameter of the circle. NM is parallel to RS and ∠MRS = 29°. Calculate : ∠RNM
Prove that the perimeter of a right triangle is equal to the sum of the diameter of its incircle and twice the diameter of its circumcircle.
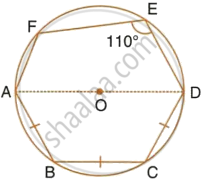
In the following figure, AD is the diameter of the circle with centre O. Chords AB, BC and CD are equal. If ∠DEF = 110°, calculate: ∠AEF
In the given figure, RS is a diameter of the circle. NM is parallel to RS and ∠MRS = 29°. Calculate : ∠NRM
In the given figure, AB is a diameter of the circle with centre O. DO is parallel to CB and ∠DCB = 120°.
Calculate : ∠DBC
Also, show that the ΔAOD is an equilateral triangle.

In the following figure, AD is the diameter of the circle with centre O. chords AB, BC and CD are equal. If ∠DEF = 110°, Calculate: ∠FAB.

In the given figure, O is the centre of the circle and ∠PBA = 45°. Calculate the value of ∠PQB.
In the given figure, BAD = 65°, ABD = 70°, BDC = 45°.
(i) Prove that AC is a diameter of the circle.
(ii) Find ACB.

