Advertisements
Advertisements
Question
In the given figure, RS is a diameter of the circle. NM is parallel to RS and ∠MRS = 29°. Calculate : ∠NRM
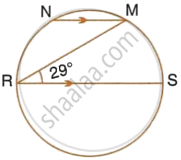
Solution
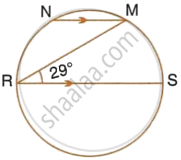
Also, RS || NM
∴ ∠NMR = ∠MRS = 29° ...(Alternate angles)
∴ ∠NMS = 90° + 29° = 119°
Also, ∠NRS + ∠MS = 180°
(Pair of opposite angles in a cyclic quadrilateral are supplementary)
`=>` ∠NMR + 29° + 119° = 180°
`=>` ∠NRM = 180° – 148°
∴ ∠NRM = 32°
APPEARS IN
RELATED QUESTIONS
Prove that the parallelogram, inscribed in a circle, is a rectangle.
Two circles intersect at P and Q. Through P diameters PA and PB of the two circles are drawn. Show that the points A, Q and B are collinear.
In the figure, given alongside, AB || CD and O is the centre of the circle. If ∠ADC = 25°; find the angle AEB. Give reasons in support of your answer.

Prove that the perimeter of a right triangle is equal to the sum of the diameter of its incircle and twice the diameter of its circumcircle.
In the figure, given below, AB and CD are two parallel chords and O is the centre. If the radius of the circle is 15 cm, fins the distance MN between the two chords of lengths 24 cm and 18 cm respectively.

In the following figure, AD is the diameter of the circle with centre O. chords AB, BC and CD are equal. If ∠DEF = 110°, Calculate: ∠FAB.

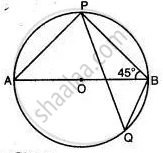
In the given figure, O is the centre of the circle and ∠PBA = 45°. Calculate the value of ∠PQB.
In Fig, Chord ED is parallel to the diameter AC of the circle. Given ∠CBE = 65°, Calculate ∠ DEC.
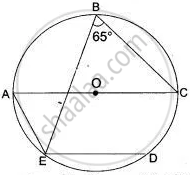
In the figure, ∠DBC = 58°. BD is diameter of the circle.
Calculate:
- ∠BDC
- ∠BEC
- ∠BAC
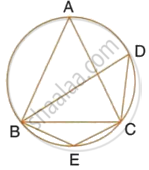
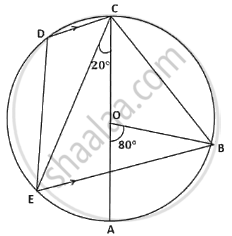
In the given figure AC is the diameter of the circle with centre O. CD is parallel to BE.
∠AOB = 80° and ∠ACE = 20°.
Calculate
- ∠BEC
- ∠BCD
- ∠CED