Advertisements
Advertisements
Question
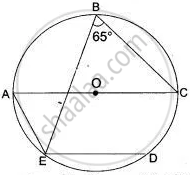
In Fig, Chord ED is parallel to the diameter AC of the circle. Given ∠CBE = 65°, Calculate ∠ DEC.
Solution
Consider the arc CDE. We find that ∠ CBE and ∠ CAE are the angles in the same segment of arc CDE.
∴ ∠ CAE = ∠ CBE
⇒ ∠ CAE = 65° ...( ∵ ∠ CBE = 65° )
Since AC is the diameter of the circle and the angle in a semicircle is a right angle.
Therefore, ∠ AEC = 90°.
Now, in Δ ACE, we have
∠ ACE + ∠ AEC + ∠ CAE = 180°
⇒ ∠ ACE + 90° + 65° = 180°
⇒ ∠ ACE = 25°
But ∠ DEC and ∠ ACE are alternate angles, because AC || DE.
∴ ∠ DEC = ∠ ACE = 25°.
APPEARS IN
RELATED QUESTIONS
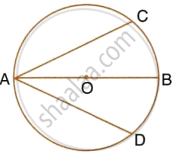
In the given figure, ∠BAD = 65°, ∠ABD = 70°, ∠BDC = 45°
1) Prove that AC is a diameter of the circle.
2) Find ∠ACB
Calculate the area of the shaded region, if the diameter of the semicircle is equal to 14 cm. Take `pi = 22/7`

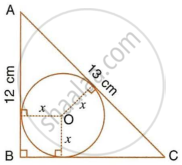
ABC is a right angles triangle with AB = 12 cm and AC = 13 cm. A circle, with centre O, has been inscribed inside the triangle.
Calculate the value of x, the radius of the inscribed circle.
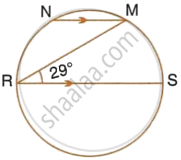
In the given figure, RS is a diameter of the circle. NM is parallel to RS and ∠MRS = 29°. Calculate : ∠RNM
ABCD is a cyclic quadrilateral in which AB is parallel to DC and AB is a diameter of the circle. Given ∠BED = 65°; calculate :
- ∠DAB,
- ∠BDC.

In the given figure, AB is a diameter of the circle with centre O. DO is parallel to CB and ∠DCB = 120°.
Calculate:
- ∠DAB,
- ∠DBA,
- ∠DBC,
- ∠ADC.
Also, show that the ΔAOD is an equilateral triangle.

Prove that the perimeter of a right triangle is equal to the sum of the diameter of its incircle and twice the diameter of its circumcircle.
In the given figure, AB is the diameter of a circle with centre O.
If chord AC = chord AD, prove that:
- arc BC = arc DB
- AB is bisector of ∠CAD.
Further, if the length of arc AC is twice the length of arc BC, find:
- ∠BAC
- ∠ABC
In the given figure, BAD = 65°, ABD = 70°, BDC = 45°.
(i) Prove that AC is a diameter of the circle.
(ii) Find ACB.

