Advertisements
Advertisements
Question
In triangle ABC; ∠ABC = 90°, ∠CAB = x°, tan x° = `(3)/(4)` and BC = 15 cm. Find the measures of AB and AC.
Solution
Consider the figure: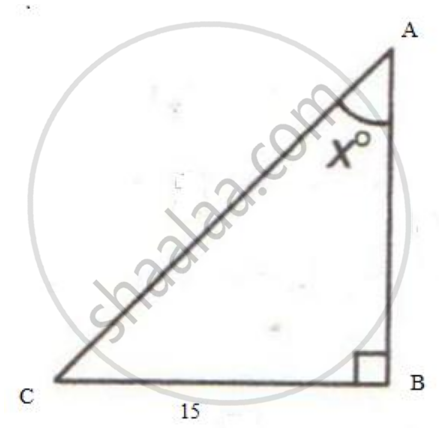
tan x° = `(3)/(4)`
i.e. `"perpendicular"/"base" = "BC"/"AB" =(3)/(4)`
Therefore if length of base = 4x, length of perpendicular = 3x
Since
BC2 + AB2 = AC2 ...[Using Pythagoras Theorem]
(3x)2 + (4x)2 = AC2
AC2 = 9x2 + 16x2
AC2 = 25x2
AC = `sqrt(25x^2)`
∴ AC = 5x
Now
BC = 15
3x = 15
x = `15/3`
x = 5
Therefore
AB = 4x
= 4 × 5
= 20 cm
And
AC = 5x
= 5 × 5
= 25 cm
APPEARS IN
RELATED QUESTIONS
If A = B = 60°. Verify `tan (A - B) = (tan A - tan B)/(1 + tan tan B)`
If 3 cot `theta = 2, `show that `((4 sin theta - 4 cos theta))/((2 sin theta + 6 cos theta ))=1/3`
If 3x = cosecθ = and `3/x= cottheta` find the value of 3`(x^2-1/x^2)`.
Prove that
cosec (65 °+ θ) sec (25° − θ) − tan (55° − θ) + cot (35° + θ) = 0
In rhombus ABCD, diagonals AC and BD intersect each other at point O.
If cosine of angle CAB is 0.6 and OB = 8 cm, find the lengths of the side and the diagonals of the rhombus.
In each of the following, one trigonometric ratio is given. Find the values of the other trigonometric.
tan C = `(5)/(12)`
If sinA = `(3)/(5)`, find cosA and tanA.
In an isosceles triangle ABC, AB = BC = 6 cm and ∠B = 90°. Find the values of cosec C
If sin A = `(7)/(25)`, find the value of : `(2"tanA")/"cot A - sin A"`
If cosec θ = `(29)/(20)`, find the value of: cosec θ - `(1)/("cot" θ)`
