Advertisements
Advertisements
Question
Point P is the midpoint of seg AB. If co-ordinates of A and B are (-4, 2) and (6, 2) respectively then find the co-ordinates of point P.
(A) (-1,2) (B) (1,2) (C) (1,-2) (D) (-1,-2)
Solution
(3) B
APPEARS IN
RELATED QUESTIONS
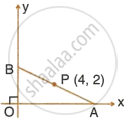
In the given figure, P(4, 2) is mid-point of line segment AB. Find the co-ordinates of A and B.
One end of the diameter of a circle is (–2, 5). Find the co-ordinates of the other end of it, if the centre of the circle is (2, –1).
A(2, 5), B(1, 0), C(−4, 3) and D(–3, 8) are the vertices of quadrilateral ABCD. Find the co-ordinates of the mid-points of AC and BD. Give a special name to the quadrilateral.
The co-ordinates of the centroid of a triangle PQR are (2, –5). If Q = (–6, 5) and R = (11, 8); calculate the co-ordinates of vertex P.
Complete the table below the graph with the help of the following graph.

| Sr. No. | First point | Second point | Co-ordinates of first point (x1 , y1) | Co-ordinates of second point (x2 , y2) | `(y_2 - y_2)/(x_2 - x_2)` |
| 1 | C | E | (1, 0) | (3,4) | `4/2=2` |
| 2 | A | B | (-1,-4) | (0,-2) | `2/1 = 2` |
| 3 | B | D | (0,-2) | (2,2) | `4/2=2` |
Find the midpoint of the line segment joining the following pair of point :
(3a-2b, Sa+7b) and (a+4b, a-3b)
P( -2, 5), Q(3, 6 ), R( -4, 3) and S(-9, 2) are the vertices of a quadrilateral. Find the coordinates of the midpoints of the diagonals PR and QS. Give a special name to the quadrilateral.
If (-3, 2), (1, -2) and (5, 6) are the midpoints of the sides of a triangle, find the coordinates of the vertices of the triangle.
Find the centroid of a triangle whose vertices are (3, -5), (-7, 4) and ( 10, -2).
A( 4, 2), B(-2, -6) and C(l, 1) are the vertices of triangle ABC. Find its centroid and the length of the median through C.
ABC is a triangle whose vertices are A(-4, 2), B(O, 2) and C(-2, -4). D. E and Fare the midpoint of the sides BC, CA and AB respectively. Prove that the centroid of the Δ ABC coincides with the centroid of the Δ DEF.
A(3, 1), B(y, 4) and C(1, x) are vertices of a triangle ABC and G(3, 4) is its centroid. Find the values of x and y. Also, find the length of side BC.
The midpoint of the line segment joining (2a, 4) and (-2, 2b) is (1, 2a+1). Find the value of a and b.
show that the points A(- 1, 2), B(2, 5) and C(- 5, – 2) are collinear.
Find the mid-point of the line segment joining the points
(8, −2) and (−8, 0)
Find the mid-point of the line segment joining the points
`(1/2, (-3)/7)` and `(3/2, (-11)/7)`
The coordinates of the point C dividing the line segment joining the points P(2, 4) and Q(5, 7) internally in the ratio 2 : 1 is
The ratio in which the x-axis divides the line segment joining the points (6, 4) and (1, −7) is
The mid-point of the line joining (−a, 2b) and (−3a, −4b) is
Find coordinates of midpoint of the segment joining points (0, 2) and (12, 14)
Find the co-ordinates of centroid of a triangle if points D(–7, 6), E(8, 5) and F(2, –2) are the mid-points of the sides of that triangle.
