Advertisements
Advertisements
Question
Solve \[\left| x + 1 \right| + \left| x \right| > 3\]
Solution
\[\text{ We have }, \left| x + 1 \right| + \left| x \right| > 3\]
\[\text{ As }, \left| x + 1 \right| = \binom{\left( x + 1 \right), x \geq - 1}{ - \left( x + 1 \right), x < - 1}\]
\[\text{ and } \left| x \right| = \binom{x, x \geq 0}{ - x, x < 0}\]
\[\text{ Case I: When } x < - 1, \]
\[\left| x + 1 \right| + \left| x \right| > 3\]
\[ \Rightarrow - \left( x + 1 \right) - x > 3\]
\[ \Rightarrow - 2x - 1 > 3\]
\[ \Rightarrow - 2x > 4\]
\[ \Rightarrow x < \frac{4}{- 2}\]
\[ \Rightarrow x < - 2\]
\[\text{ So }, x \in \left( - \infty , - 2 \right)\]
\[\text{ Case II: When } - 1 \leq x < 0, \]
\[\left| x + 1 \right| + \left| x \right| > 3\]
\[ \Rightarrow \left( x + 1 \right) - x > 3\]
\[ \Rightarrow 1 > 3, \text{ which is not possible }\]
\[\text{ So }, x \in \phi\]
\[\text{ Case III: When x } \geq 0, \]
\[\left| x + 1 \right| + \left| x \right| > 3\]
\[ \Rightarrow \left( x + 1 \right) + x > 3\]
\[ \Rightarrow 2x + 1 > 3\]
\[ \Rightarrow 2x > 2\]
\[ \Rightarrow x > \frac{2}{2}\]
\[ \Rightarrow x > 1\]
\[\text{ So }, x \in \left( 1, \infty \right)\]
\[\text{ From all the cases, we get }\]
\[x \in \left( - \infty , - 2 \right) \cup \left( 1, \infty \right)\]
APPEARS IN
RELATED QUESTIONS
Solve: −4x > 30, when x ∈ R
Solve: −4x > 30, when x ∈ Z
3x + 9 ≥ −x + 19
\[2\left( 3 - x \right) \geq \frac{x}{5} + 4\]
\[\frac{4 + 2x}{3} \geq \frac{x}{2} - 3\]
\[x - 2 \leq \frac{5x + 8}{3}\]
\[\frac{4x + 3}{2x - 5} < 6\]
\[\frac{5x + 8}{4 - x} < 2\]
\[\frac{x - 1}{x + 3} > 2\]
Solve each of the following system of equations in R.
2x − 7 > 5 − x, 11 − 5x ≤ 1
Solve each of the following system of equations in R.
4x − 1 ≤ 0, 3 − 4x < 0
Solve each of the following system of equations in R.
2 (x − 6) < 3x − 7, 11 − 2x < 6 − x
Solve
\[\left| \frac{3x - 4}{2} \right| \leq \frac{5}{12}\]
Solve \[\frac{1}{\left| x \right| - 3} < \frac{1}{2}\]
Solve
\[\left| \frac{2x - 1}{x - 1} \right| > 2\]
Solve \[\left| x - 1 \right| + \left| x - 2 \right| + \left| x - 3 \right| \geq 6\]
Solve \[\frac{\left| x - 2 \right| - 1}{\left| x - 2 \right| - 2} \leq 0\]
Solve \[1 \leq \left| x - 2 \right| \leq 3\]
Mark the correct alternative in each of the following:
If x is a real number and \[\left| x \right|\]\[<\]5, then
Mark the correct alternative in each of the following:
If x and a are real numbers such that a\[>\]0 and \\left| x \right|\]\[>\]a, then
Mark the correct alternative in each of the following:
\[\left| x - 1 \right|\]\[>\]5, then
Mark the correct alternative in each of the following:
The inequality representing the following graph is
Mark the correct alternative in each of the following:
The linear inequality representing the solution set given in 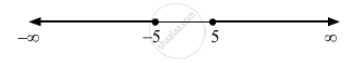
Mark the correct alternative in each of the following:
If \[\left| x + 3 \right|\]\[\geq\]10, then
Solve the inequality, 3x – 5 < x + 7, when x is an integer.
Solve 1 ≤ |x – 2| ≤ 3.
Solve for x, |x + 1| + |x| > 3.
Solve for x, `(|x + 3| + x)/(x + 2) > 1`.
If a < b and c < 0, then `a/c` ______ `b/c`.
If |x − 1| ≤ 2, then –1 ______ x ______ 3
Solve for x, the inequality given below.
`-5 ≤ (2 - 3x)/4 ≤ 9`
Solve for x, the inequality given below.
4x + 3 ≥ 2x + 17, 3x – 5 < –2
A solution of 9% acid is to be diluted by adding 3% acid solution to it. The resulting mixture is to be more than 5% but less than 7% acid. If there is 460 litres of the 9% solution, how many litres of 3% solution will have to be added?
In drilling world’s deepest hole it was found that the temperature T in degree celcius, x km below the earth’s surface was given by T = 30 + 25(x – 3), 3 ≤ x ≤ 15. At what depth will the temperature be between 155°C and 205°C?
If – 4x ≥ 12, then x ______ – 3.
If p > 0 and q < 0, then p – q ______ p.
If |x + 2| > 5, then x ______ – 7 or x ______ 3.
