Advertisements
Advertisements
Question
The following table gives the activities of a project and their duration in days
| Activity | 1 - 2 | 1 - 3 | 2 - 3 | 2 - 4 | 3 - 4 | 3 - 5 | 4 - 5 |
| Duration | 5 | 8 | 6 | 7 | 5 | 4 | 8 |
Construct the network and calculate the earliest start time, earliest finish time, latest start time and latest finish time of each activity and determine the Critical path of the project and duration to complete the project.
Solution
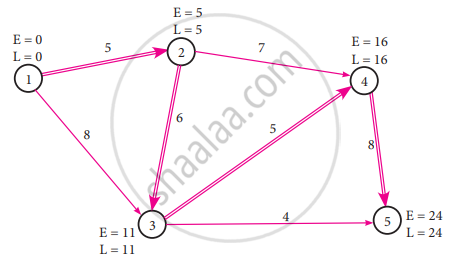
E1 = 0
E2 = 0 + 5 = 5
E3 = (0 + 8) or (5 + 6)
Whichever is maximum
= 11
E4 = (11 + 5) or (5 + 7)
Whichever is maximum
= 16
E5 = (11 + 4) or (16 + 8)
Whichever is maximum
= 24
L5 = 24
L4 = 24 – 8 = 16
L3 = (24 – 4) or (16 – 5)
whichever is minimum
= 11
L2 = (16 – 6) or (16 – 7)
whichever is minimum
= 5
L1 = (5 – 5) or (11 – 8)
whichever is minimum
L1 = 0
| Activity | Duration tij |
EST | EFT = EST + tij | LST = LFT – tij | LFT |
| 1 - 2 | 5 | 0 | 5 | 5 – 5 = 0 | 5 |
| 1 - 3 | 8 | 0 | 8 | 11 – 8 = 3 | 11 |
| 2 - 3 | 6 | 5 | 11 | 11 – 6 = 5 | 11 |
| 2 - 4 | 7 | 5 | 12 | 16 – 7 = 9 | 16 |
| 3 - 4 | 5 | 11 | 16 | 16 – 5 = 11 | 16 |
| 3 - 5 | 4 | 11 | 15 | 24 – 4 = 20 | 24 |
| 4 - 5 | 8 | 16 | 24 | 24 – 8 = 16 | 24 |
Since EFT and LFT are same in 1 - 2, 2 - 3, 3 - 4 and 4 - 5.
Hence the critical path is 1 - 2 - 3 - 4 - 5 and the duration time taken is 24 days.
APPEARS IN
RELATED QUESTIONS
Draw the network for the project whose activities with their relationships are given below:
Activities A, D, E can start simultaneously; B, C > A; G, F > D, C; H > E, F.
Construct the network for each the projects consisting of various activities and their precedence relationships are as given below:
| Activity | A | B | C | D | E | F | G | H | I | J | K |
| Immediate Predecessors | - | - | - | A | B | B | C | D | E | H, I | F, G |
Construct the network for the project whose activities are given below.
| Activity | 0 - 1 | 1 - 2 | 1 - 3 | 2 - 4 | 2 - 5 | 3 - 4 | 3 - 6 | 4 - 7 | 5 - 7 | 6 - 7 |
| Duration (in week) | 3 | 8 | 12 | 6 | 3 | 3 | 8 | 5 | 3 | 8 |
Calculate the earliest start time, earliest finish time, latest start time and latest finish time of each activity. Determine the critical path and the project completion time.
The following table use the activities in a construction projects and relevant information
| Activity | 1 - 2 | 1 - 3 | 2 - 3 | 2 - 4 | 3 - 4 | 4 - 5 |
| Duration (in days) |
22 | 27 | 12 | 14 | 6 | 12 |
Draw the network for the project, calculate the earliest start time, earliest finish time, latest start time and latest finish time of each activity and find the critical path. Compute the project duration.
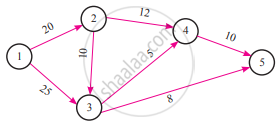
The critical path of the following network is
In a network while numbering the events which one of the following statements is false?
Which of the following is not correct?
The objective of network analysis is to
Draw the network diagram for the following activities.
| Activity code | A | B | C | D | E | F | G |
| Predecessor activity | - | - | A | A | B | C | D, E |
The following table gives the characteristics of the project
| Activity | 1 - 2 | 1 - 3 | 2 - 3 | 3 - 4 | 3 - 5 | 4 - 6 | 5 - 6 | 6 - 7 |
| Duration (in days) |
5 | 10 | 3 | 4 | 6 | 6 | 5 | 5 |
Draw the network for the project, calculate the earliest start time, earliest finish time, latest start time and latest finish time of each activity and find the critical path. Compute the project duration.
