Advertisements
Advertisements
Question
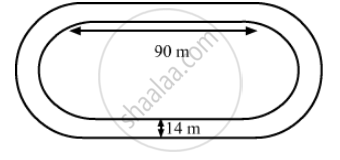
The inside perimeter of a running track (shown in the following figure) is 400 m. The length of each of the straight portion is 90 m and the ends are semi-circles. If the track is everywhere 14 m wide. find the area of the track. Also find the length of the outer running track.
Solution
It is given that, `"length of each straight portion"`and `"width of track=14m"`
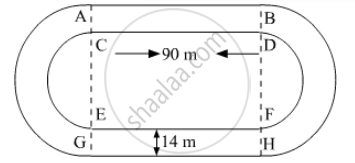
We know that the circumference C of semicircle of radius be r is \[C = \pi r\]
The inside perimeter of running track is the sum of twice the length of straight portion and circumferences of semicircles. So,
inside perimeter of running track = 400 m
\[2l + 2\pi r = 400 m\]
\[\Rightarrow 2 \times 90 + 2 \times \frac{22}{7} \times r = 400 m\]
\[\Rightarrow 2 \times 90 + 2 \times \frac{22}{7} \times r = 400 m\]
Thus, radius of inner semicircle is 35 m.
Now,
radius of outer semi circle r' = 35 + 14 = 49 m
Area of running track =\[2 \times \text{ Area of rectangle } + 2 \times \text{ Area of outer semi circle } - 2 \times \text{ Area of inner semicircle }\]
\[= 2 \times 90 \times 14 + 2 \times \frac{\pi(49 )^2}{2} - 2 \times \frac{\pi(35 )^2}{2}\]
\[= 2520 + \pi \times \left( 49 + 35 \right)\left( 49 - 35 \right)\]
\[= 2520 + \pi \times \left( 49 + 35 \right)\left( 49 - 35 \right)\]
\[= 2520 + 3696 = 6216 m^2\]
Hence, the area of running track = 6216 m2
Now, length L of outer running track isL = 2 × l + 2 \[\pi\]
\[= 2 \times 90 + 2\pi \times 49\]
\[= 180 + 2 \times \frac{22}{7} \times 49\]
\[= 180 + 308 = 488 m\]
Hence, the length L of outer running track is 488 m.
APPEARS IN
RELATED QUESTIONS
One side of a rectangle is 12 cm long and its diagonal measure 37 cm. Find the other side and the area of the rectangle.
In the following figure, OACB is a quadrant of a circle with centre O and radius 3.5 cm. If OD = 2 cm, find the area of the (i) quadrant OACB (ii) shaded region.

In the following figure, there are three semicircles, A, B and C having diameter 3 cm each, and another semicircle E having a circle D with diameter 4.5 cm are shown. Calculate:
(i) the area of the shaded region
(ii) the cost of painting the shaded region at the rate of 25 paise per cm2 , to the nearest rupee.

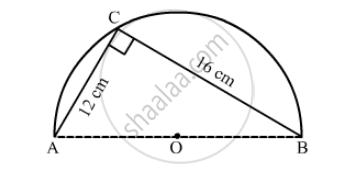
In the following figure, O is the centre of a circular arc and AOB is a straight line. Find the perimeter and the area of the shaded region correct to one decimal place. (Take π = 3.142)
The radius of a wheel is 0.25 m. The number of revolutions it will make to travel a distance of 11 km will be
Area of the largest triangle that can be inscribed in a semi-circle of radius r units is ______.
ABCD is a field in the shape of a trapezium, AD || BC, ∠ABC = 90° and ∠ADC = 60°. Four sectors are formed with centres A, B, C and D, as shown in the figure. The radius of each sector is 14 m. Find the following:
- total area of the four sectors,
- area of the remaining portion, given that AD = 55 m, BC = 45 m and AB = 30 m.

On decreasing the radius of a circle by 30%, its area is decreased by
The area of circle is equal to the sum of the areas of two circles of radii 24 cm and 7 cm. The diameter of the new circle is
The minute hand of a clock is 12 cm long. Find the area of the face of the clock described by the minute hand in 35 minutes.
