Question

Two concentric circles are of diameters 30 cm and 18 cm. Find the length of the chord of the larger circle which touches the smaller circle.

Answer 1

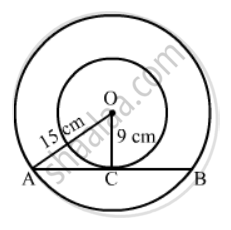
Let O be the common centre of the two circles and AB be the chord of the larger circle which touches the smaller circle at C.

Join OA and OC. Then,

OC = \[\frac{18}{2}\]

 cm = 9 cm and OA =\[\frac{30}{2}\] cm = 15 cm

We know that the tangent at any point of a circle is perpendicular to the radius through the point of contact. Also, the perpendicular drawn from the centre of a circle to a chord bisects the chord.

∴ OC ⊥ AB and C is the mid-point of AB.

In right ∆OCA,

\[{OA}^2 = {OC}^2 + {AC}^2 \left( \text{Pythagoras theorem} \right)\]
\[ \Rightarrow {AC}^2 = {OA}^2 - {OC}^2 \]
\[ \Rightarrow AC = \sqrt{{15}^2 - 9^2}\]
\[ \Rightarrow AC = \sqrt{225 - 81} = \sqrt{144} = 12 cm\]

∴ AB = 2AC = 2 × 12 cm = 24 cm

Thus, the required length of the chord is 24 cm.