Advertisements
Advertisements
Question
Two ideal gases have the same value of Cp / Cv = γ. What will be the value of this ratio for a mixture of the two gases in the ratio 1 : 2?
Advertisements
Solution
For the first ideal gas,
Cp1 = specific heat at constant pressure
Cv1 = specific heat at constant volume
n1 = number of moles of the gas
`"C"_("p"1)/"C"_("v"1) = gamma and "C"_("p"1) -"C"_("v"1) = "R"`
`=> gamma "C"_("v"1) - "C"_("v"1) ="R"`
`=> "C"_("v"1)(gamma-1) ="R"`
`=> "C"_("v"1) = "R"/(gamma -1)`
`"C"_("p"1) = gamma "R"/((gamma-1))`
For the second ideal gas,
Cp2 = specific heat at constant pressure
Cv2 = specific heat at constant volume
n2 = number of moles of the gas
`"C"_("p"2)/"C"_("v"2) = gamma and "C"_("p"2) -"C"_("v"2) = "R"`
`=> gamma "C"_("v"2) - "C"_("v"2) ="R"`
`=> "C"_("v"2)(gamma-1) ="R"`
`=> "C"_("v"2) = "R"/(gamma -1)`
`"C"_("p"2) = gamma "R"/((gamma-1))`
Given:
n1 = n2 = 1 : 2
dU1 = nCv1dt
dU2= 2nCv2dT
When the gases are mixed,
nCv1dT + 2nCv2dT = 3nCvdT
`"C"_"v" = ("C"_("v"1) +2"C"_("v"2))/3`
`= (" R"/(gamma-1) +(2"R")/(gamma-1))/3`
`= (3"R")/((gamma-1)3) = "R"/(gamma-1)`
Hence, Cp / Cv in the mixture is γ.
APPEARS IN
RELATED QUESTIONS
Keeping the number of moles, volume and temperature the same, which of the following are the same for all ideal gases?
Consider the quantity \[\frac{MkT}{pV}\] of an ideal gas where M is the mass of the gas. It depends on the
Find the number of molecules in 1 cm3 of an ideal gas at 0°C and at a pressure of 10−5mm of mercury.
Use R = 8.31 J K-1 mol-1
Let Q and W denote the amount of heat given to an ideal gas and the work done by it in an isothermal process.
Let Q and W denote the amount of heat given to an ideal gas and the work done by it in an adiabatic process.
(a) Q = 0
(b) W = 0
(c) Q = W
(d) Q ≠ W
A vessel containing one mole of a monatomic ideal gas (molecular weight = 20 g mol−1) is moving on a floor at a speed of 50 m s−1. The vessel is stopped suddenly. Assuming that the mechanical energy lost has gone into the internal energy of the gas, find the rise in its temperature.
The figure shows a cylindrical container containing oxygen (γ = 1.4) and closed by a 50-kg frictionless piston. The area of cross-section is 100 cm2, atmospheric pressure is 100 kPa and g is 10 m s−2. The cylinder is slowly heated for some time. Find the amount of heat supplied to the gas if the piston moves out through a distance of 20 cm.
The ratio of the molar heat capacities of an ideal gas is Cp/Cv = 7/6. Calculate the change in internal energy of 1.0 mole of the gas when its temperature is raised by 50 K (a) keeping the pressure constant (b) keeping the volume constant and (c) adiaba
An amount Q of heat is added to a monatomic ideal gas in a process in which the gas performs a work Q/2 on its surrounding. Find the molar heat capacity for the process
An ideal gas is taken through a process in which the pressure and the volume are changed according to the equation p = kV. Show that the molar heat capacity of the gas for the process is given by `"C" ="C"_"v" +"R"/2.`
The volume of an ideal gas (γ = 1.5) is changed adiabatically from 4.00 litres to 3.00 litres. Find the ratio of (a) the final pressure to the initial pressure and (b) the final temperature to the initial temperature.
Consider a given sample of an ideal gas (Cp/Cv = γ) having initial pressure p0 and volume V0. (a) The gas is isothermally taken to a pressure p0/2 and from there, adiabatically to a pressure p0/4. Find the final volume. (b) The gas is brought back to its initial state. It is adiabatically taken to a pressure p0/2 and from there, isothermally to a pressure p0/4. Find the final volume.
1 litre of an ideal gas (γ = 1.5) at 300 K is suddenly compressed to half its original volume. (a) Find the ratio of the final pressure to the initial pressure. (b) If the original pressure is 100 kPa, find the work done by the gas in the process. (c) What is the change in internal energy? (d) What is the final temperature? (e) The gas is now cooled to 300 K keeping its pressure constant. Calculate the work done during the process. (f) The gas is now expanded isothermally to achieve its original volume of 1 litre. Calculate the work done by the gas. (g) Calculate the total work done in the cycle.
A cubic vessel (with faces horizontal + vertical) contains an ideal gas at NTP. The vessel is being carried by a rocket which is moving at a speed of 500 ms–1 in vertical direction. The pressure of the gas inside the vessel as observed by us on the ground ______.
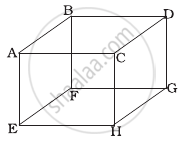
1 mole of an ideal gas is contained in a cubical volume V, ABCDEFGH at 300 K (Figure). One face of the cube (EFGH) is made up of a material which totally absorbs any gas molecule incident on it. At any given time ______.
ABCDEFGH is a hollow cube made of an insulator (Figure). Face ABCD has positive charge on it. Inside the cube, we have ionized hydrogen. The usual kinetic theory expression for pressure ______.
- will be valid.
- will not be valid since the ions would experience forces other than due to collisions with the walls.
- will not be valid since collisions with walls would not be elastic.
- will not be valid because isotropy is lost.
Diatomic molecules like hydrogen have energies due to both translational as well as rotational motion. From the equation in kinetic theory `pV = 2/3` E, E is ______.
- the total energy per unit volume.
- only the translational part of energy because rotational energy is very small compared to the translational energy.
- only the translational part of the energy because during collisions with the wall pressure relates to change in linear momentum.
- the translational part of the energy because rotational energies of molecules can be of either sign and its average over all the molecules is zero.
The container shown in figure has two chambers, separated by a partition, of volumes V1 = 2.0 litre and V2 = 3.0 litre. The chambers contain µ1 = 4.0 and µ2 = 5.0 moles of a gas at pressures p1 = 1.00 atm and p2 = 2.00 atm. Calculate the pressure after the partition is removed and the mixture attains equilibrium.
| V1 | V2 |
| µ1, p1 | µ2 |
| p2 |
