Advertisements
Advertisements
Question
Two tangent segments PA and PB are drawn to a circle with center O such that ∠APB =120°. Prove that OP = 2AP
Solution
A + P
OP bisects ∠APB
∠APO = ∠OPB =`1/2`∠𝐴𝑃𝐵 =`1/2`× 120° = 60°
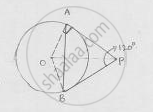
At point A
OA ⊥ AP, ∠OAP = 90°
In ΔPDA, cos 60° =`(AP)/(DP)`
`1/2=(AP)/(DP)`⇒ 𝐷𝑃 = 2𝐴𝑃
APPEARS IN
RELATED QUESTIONS
If PT is a tangent at T to a circle whose center is O and OP = 17 cm, OT = 8 cm. Find the length of tangent segment PT.
From an external point P, tangents PA and PB are drawn to a circle with center O. If CD is the tangent to the circle at a point E and PA = 14cm, find the perimeter of ΔPCD.

One chord of a circle is known to be 10 cm. The radius of this circle must be
In the given figure, a ∆ABC is drawn to circumscribe a circle of radius 4 cm such that the segments BD and DC are of lengths 8 cm and 6 cm respectively. Find the lengths of sides AB and AC, when area of ∆ABC is 84 cm2.

In the given circle with diameter AB, find the value of x.
ABC is a triangle with AB = 10 cm, BC = 8 cm and AC = 6 cm (not drawn to scale). Three circles are drawn touching each other with the vertices as their centres. Find the radii of the three circles.

State, if the following statement is true or false:
The longest chord of a circle is its diameter.
In a circle, AB and CD are two parallel chords with centre O and radius 10 cm such that AB = 16 cm and CD = 12 cm determine the distance between the two chords?
In the figure, O is the centre of a circle, AB is a chord, and AT is the tangent at A. If ∠AOB = 100°, then ∠BAT is equal to ______

A point P is 10 cm from the center of a circle. The length of the tangent drawn from P to the circle is 8 cm. The radius of the circle is equal to ______
