English Medium
Academic Year: 2015-2016
Date & Time: 19th March 2016, 10:30 am
Duration: 3h
Advertisements
From an external point P, tangents PA and PB are drawn to a circle with centre O. If ∠PAB = 50°, then find ∠AOB.
Chapter: [0.042] Circles
In Fig. 1, AB is a 6 m high pole and CD is a ladder inclined at an angle of 60° to the horizontal and reaches up to a point D of pole. If AD = 2.54 m, find the length of the ladder. (use3√=1.73)
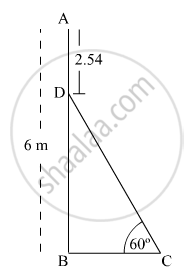
Chapter: [0.053] Some Applications of Trigonometry
Find the 9th term from the end (towards the first term) of the A.P. 5, 9, 13, ...., 185
Chapter: [0.024] Arithmetic Progressions
Cards marked with number 3, 4, 5, ...., 50 are placed in a box and mixed thoroughly. A card is drawn at random form the box. Find the probability that the selected card bears a perfect square number.
Chapter: [0.07200000000000001] Probability [0.07200000000000001] Probability
If `x=2/3` and x =−3 are roots of the quadratic equation ax2 + 7x + b = 0, find the values of a and b.
Chapter: [0.023] Quadratic Equations
Find the ratio in which y-axis divides the line segment joining the points A(5, –6) and B(–1, –4). Also find the coordinates of the point of division.
Chapter: [0.031] Lines (In Two-dimensions)
In Fig. 2, a circle is inscribed in a ΔABC, such that it touches the sides AB, BC and CA at points D, E and F respectively. If the lengths of sides AB, BC and CA and 12 cm, 8 cm and 10 cm respectively, find the lengths of AD, BE and CF.
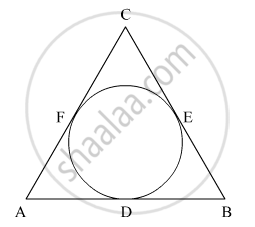
Chapter: [0.061] Areas Related to Circles
The x-coordinate of a point P is twice its y-coordinate. If P is equidistant from Q(2, –5) and R(–3, 6), find the coordinates of P.
Chapter: [0.031] Lines (In Two-dimensions)
How many terms of the A.P. 18, 16, 14, .... be taken so that their sum is zero?
Chapter: [0.024] Arithmetic Progressions
In Fig. 3, AP and BP are tangents to a circle with centre O, such that AP = 5 cm and ∠APB = 60°. Find the length of chord AB.
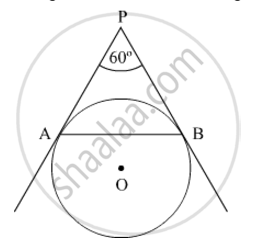
Chapter: [0.061] Areas Related to Circles
Advertisements
In Fig. 4, ABCD is a square of side 14 cm. Semi-circles are drawn with each side of square as diameter. Find the area of the shaded region.
(use `pi=22/7`)
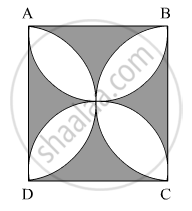
Chapter: [0.061] Areas Related to Circles
In Fig. 5, is a decorative block, made up two solids – a cube and a hemisphere. The base of the block is a cube of side 6 cm and the hemisphere fixed on the top has diameter of 3.5 cm. Find the total surface area of the bock `(Use pi=22/7)`
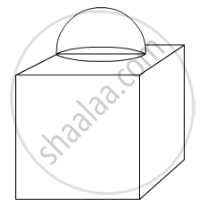
Chapter: [0.062] Surface Areas and Volumes
In Fig. 6, ABC is a triangle coordinates of whose vertex A are (0, −1). D and E respectively are the mid-points of the sides AB and AC and their coordinates are (1, 0) and (0, 1) respectively. If F is the mid-point of BC, find the areas of ∆ABC and ∆DEF.

Chapter: [0.031] Lines (In Two-dimensions)
In Fig. 7, are shown two arcs PAQ and PBQ. Arc PAQ is a part of circle with centre O and radius OP while arc PBQ is a semi-circle drawn on PQ ad diameter with centre M. If OP = PQ = 10 cm show that area of shaded region is `25(sqrt3-pi/6)cm^2`.

Chapter: [0.061] Areas Related to Circles
If the sum of first 7 terms of an A.P. is 49 and that of its first 17 terms is 289, find the sum of first n terms of the A.P.
Chapter: [0.024] Arithmetic Progressions
Solve for x
`(2x)/(x-3)+1/(2x+3)+(3x+9)/((x-3)(2x+3)) = 0, x!=3,`
Chapter: [0.023] Quadratic Equations
A well of diameter 4 m is dug 21 m deep. The earth taken out of it has been spread evenly all around it in the shape of a circular ring of width 3 m to form an embankment. Find the height of the embankment.
Chapter: [0.062] Surface Areas and Volumes
The sum of the radius of base and height of a solid right circular cylinder is 37 cm. If the total surface area of the solid cylinder is 1628 sq. cm, find the volume of the cylinder. `("use " pi=22/7)`
Chapter: [0.062] Surface Areas and Volumes
The angles of depression of the top and bottom of a 50 m high building from the top of a tower are 45° and 60° respectively. Find the height of the tower and the horizontal distance between the tower and the building (use `sqrt3`=1.73)
Chapter: [0.053] Some Applications of Trigonometry
In a single throw of a pair of different dice, what is the probability of getting (i) a prime number on each dice?
Chapter: [0.07200000000000001] Probability [0.07200000000000001] Probability
In a single throw of a pair of different dice, what is the probability of getting a total of 9 or 11?
Chapter: [0.07200000000000001] Probability [0.07200000000000001] Probability
Advertisements
A passenger, while boarding the plane, slipped form the stairs and got hurt. The pilot took the passenger in the emergency clinic at the airport for treatment. Due to this, the plane got delayed by half an hour. To reach the destination 1500 km away in time, so that the passengers could catch the connecting flight, the speed of the plane was increased by 250 km/hour than the usual speed. Find the usual speed of the plane
What value is depicted in this question?
Chapter: [0.053] Some Applications of Trigonometry
Prove that the lengths of the tangents drawn from an external point to a circle are equal.
Chapter: [0.042] Circles
Draw two concentric circles of radii 3 cm and 5 cm. Construct a tangent to smaller circle from a point on the larger circle. Also measure its length.
Chapter: [0.033] Constructions
In Fig. 8, O is the centre of a circle of radius 5 cm. T is a point such that OT = 13 cm and OT intersects circle at E. If AB is a tangent to the circle at E, find the length of AB, where TP and TQ are two tangents to the circle.

Chapter: [0.042] Circles
Find x in terms of a, b and c: `a/(x-a)+b/(x-b)=(2c)/(x-c)`
Chapter: [0.023] Quadratic Equations
A bird is sitting on the top of a 80 m high tree. From a point on the ground, the angle of elevation of the bird is 45°. The bird flies away horizontally in such a way that it remained at a constant height from the ground. After 2 seconds, the angle of elevation of the bird from the same point is 30°. Find the speed of flying of the bird.
`("Take"sqrt3=1.732)`
Chapter: [0.053] Some Applications of Trigonometry
A thief runs with a uniform speed of 100 m/minute. After one minute, a policeman runs after the thief to catch him. He goes with a speed of 100 m/minute in the first minute and increases his speed by 10 m/minute every succeeding minute. After how many minutes the policeman will catch the thief.
Chapter: [0.022000000000000002] Pair of Linear Equations in Two Variables
Prove that the area of a triangle with vertices (t, t −2), (t + 2, t + 2) and (t + 3, t) is independent of t.
Chapter: [0.031] Lines (In Two-dimensions)
A game of chance consists of spinning an arrow on a circular board, divided into 8 equal parts, which comes to rest pointing at one of the numbers 1, 2, 3, ..., 8 (Fig. 9), which are equally likely outcomes. What is the probability that the arrow will point at (i) an odd number (ii) a number greater than 3 (iii) a number less than 9.
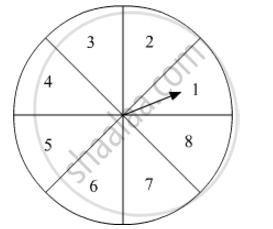
Chapter: [0.07200000000000001] Probability [0.07200000000000001] Probability
An elastic belt is placed around the rim of a pulley of radius 5 cm. (Fig. 10) From one point C on the belt, the elastic belt is pulled directly away from the centre O of the pulley until it is at P, 10 cm from the point O. Find the length of the belt that is still in contact with the pulley. Also find the shaded area (use π = 3.14 and `sqrt3=1.73)`

Chapter: [0.061] Areas Related to Circles
A bucket open at the top is in the form of a frustum of a cone with a capacity of 12308.8 cm3. The radii of the top and bottom circular ends are 20 cm and 12 cm, respectively. Find the height of the bucket and the area of metal sheet used in making the bucket. (use π = 3.14)
Chapter: [0.053] Some Applications of Trigonometry
Other Solutions
Submit Question Paper
Help us maintain new question papers on Shaalaa.com, so we can continue to help studentsonly jpg, png and pdf files
CBSE previous year question papers Class 10 Mathematics with solutions 2015 - 2016
Previous year Question paper for CBSE Class 10 Maths-2016 is solved by experts. Solved question papers gives you the chance to check yourself after your mock test.
By referring the question paper Solutions for Mathematics, you can scale your preparation level and work on your weak areas. It will also help the candidates in developing the time-management skills. Practice makes perfect, and there is no better way to practice than to attempt previous year question paper solutions of CBSE Class 10.
How CBSE Class 10 Question Paper solutions Help Students ?
• Question paper solutions for Mathematics will helps students to prepare for exam.
• Question paper with answer will boost students confidence in exam time and also give you an idea About the important questions and topics to be prepared for the board exam.
• For finding solution of question papers no need to refer so multiple sources like textbook or guides.
