(English Medium)
Academic Year: 2011-2012
Date: March 2012
Advertisements
Attempt all Question From Question 1 to Question 4
Attempt Any Four From Question 5 to Question 11
If A = `[(3, 1),(-1, 2)]` and I = `[(1, 0),(0, 1)]`, find A2 – 5A + 7I.
Chapter: [0.09] Matrices
The monthly pocket money of Ravi and Sanjeev are in the ratio 5 : 7. Their expenditures are in the ratio 3 : 5. If each saves Rs. 80 every month, find their monthly pocket money.
Chapter: [0.07] Ratio and Proportion
Using the Remainder Theorem, factorise the following completely:
3x3 + 2x2 – 19x + 6
Chapter: [0.08] Factorization
On what sum of money will the difference between the compound interest and simple interest for 2 years be equal to Rs. 25 if the rate of interest charged for both is 5% p.a.?
Chapter: [0.01] Compound Interest
ABC is an isosceles right-angled triangle with ∠ABC = 90°. A semi-circle is drawn with AC as the diameter. If AB = BC = 7 cm, find the area of the shaded region. [Take π = 22/7]
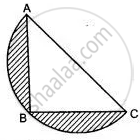
Chapter: [0.04] Mensuration [0.18] Constructions
Given a line segment AB joining the points A(–4, 6) and B(8, –3). Find
1) The ratio in which AB is divided by y-axis.
2) Find the coordinates of the point of intersection.
3) The length of AB.
Chapter: [0.13] Co-ordinate Geometry Distance and Section Formula
In the given figure O is the centre of the circle and AB is a tangent at B. If AB = 15 cm and AC = 7.5 cm. Calculate the radius of a circle.

Chapter: [0.17] Circles
Evaluate without using trigonometric tables:
`cos^2 26^@ + cos 64^@ sin 26^@ + (tan 36^@)/(cot 54^@)`
Chapter: [0.05] Trigonometry
Marks obtained by 40 students in a short assessment is given below, where a and b are two missing data.
| Marks | 5 | 6 | 7 | 8 | 9 |
| Number of Students | 6 | a | 16 | 13 | b |
If the mean of the distribution is 7.2, find a and b.
Chapter: [0.06] Statistics
Kiran deposited Rs. 200 per month for 36 months in a bank’s recurring deposit account. If the bank pays interest at the rate of 11% per annum, find the amount she gets on maturity.
Chapter: [0.02] Banking
Advertisements
Two coins are tossed once. Find the probability of getting
1) 2 heads
2) at least 1 tail.
Chapter: [0.07] Probability
Using graph paper and taking 1 cm = 1 unit along both x-axis and y-axis.
1) Plot the points A(–4, 4) and B(2, 2).
2) Reflect A and B in the origin to get the images A’ and B’ respectively.
3) Write down the coordinates of A’ and B’.
4) Give the geometrical name for the figure ABA’B’.
5) Draw and name its lines of symmetry.
Chapter: [0.04] Symmetry
In the given figure, AB is the diameter of a circle with centre O. ∠BCD = 130o. Find:
1) ∠DAB
2) ∠DBA

Chapter: [0.17] Circles
Given `[(2, 1),(-3,4)]` . X = `[(7),(6)]`. Write:
- the order of the matrix X.
- the matrix X.
Chapter: [0.09] Matrices
A page from the Savings Bank Account of Mr Prateek is given below:
| Date | Particulars | Withdrawal (In Rs.) |
Deposit (In Rs.) |
Balances (In Rs.) |
| January 1st, 2006 | B/F | - | - | 1270 |
| January 7th, 2006 | By Cheque | - | 2310 | 3580 |
| March 9th, 2006 | To Self | 2000 | - | 1580 |
| June 26th, 2006 | By Cash | - | 6200 | 7780 |
| June 10th 2006 | To Cheque | 4500 | - | 3280 |
| July 15th, 2006 | By Clearing | - | 2630 | 5910 |
| October 18th, 2006 | To Cheque | 530 | - | 5380 |
| October 27th, 2006 | To Self | 2690 | - | 2690 |
| November 3rd, 2006 | By Cash | - | 1500 | 4190 |
| December 6th, 2006 | To Cheque | 950 | - | 3240 |
| December 23rd, 2006 | By Transfer | - | 2920 | 6260 |
If he receives Rs. 198 as interest on 1st January 2007, find the rate of interest paid by the bank.
Chapter: [0.02] Banking
The printed price of an article is ₹ 60000. The wholesaler allows a discount of 20% to the shopkeeper. The shopkeeper sells the article to the customer at the printed price. Sales tax (under VAT) is charged at the rate of 6% at every stage. Find :
(i) the cost to the shopkeeper inclusive of tax.
(ii) VAT paid by the shopkeeper to the Government.
(iii) the cost to the customer inclusive of tax.
Chapter: [0.01] GST (Goods and Services Tax)
Solve the following inequation and represent the solution set on the number line:
4x - 19 < (3x)/5 - 2 <= (-2)/5 + x, x ∈ R
Chapter: [0.04] Linear Inequations
Without solving the following quadratic equation, find the value of m for which the given equation has equation has real and equal roots.
`x^2 + 2(m - 1)x + (m + 5) = 0`
Chapter: [0.05] Quadratic Equations
A hollow sphere of internal and external radii 6 cm and 8 cm respectively is melted and recast into small cones of base radius 2 cm and height 8 cm. Find the number of cones.
Chapter: [0.04] Mensuration [0.04] Mensuration
Solve the following equation and give your answer correct to 3 significant figures:
5x2 – 3x – 4 = 0
Chapter: [0.05] Quadratic Equations
As observed from the top of an 80 m tall lighthouse, the angles of depression of two ships on the same side of the lighthouse of the horizontal line with its base are 30° and 40° respectively. Find the distance between the two ships. Give your answer correct to the nearest meter.
Chapter: [0.05] Trigonometry
Advertisements
A man invests Rs. 9600 on Rs. 100 shares at Rs. 80. If the company pays him 18%
dividend find:
(1) The number of shares he buys.
(2) His total dividend.
(3) His percentage return on the shares
Chapter: [0.03] Shares and Dividends
In the given figure ΔABC and ΔAMP are right angled at B and M respectively. Given AC = 10 cm, AP = 15 cm and PM = 12 cm.

1) Prove ΔABC ~ ΔAMP
2) Find AB and BC.
Chapter: [0.15] Similarity
if x = `(sqrt(a + 1) + sqrt(a-1))/(sqrt(a + 1) - sqrt(a - 1))` using properties of proportion show that `x^2 - 2ax + 1 = 0`
Chapter: [0.07] Ratio and Proportion
The line through A(–2, 3) and B(4, b) is perpendicular to the line 2x – 4y = 5. Find the value of b.
Chapter: [0.14] Co-ordinate Geometry Equation of a Line
Prove that `(tan^2 theta)/(sec theta - 1)^2 = (1 + cos theta)/(1 - cos theta)`
Chapter: [0.05] Trigonometry
A car covers a distance of 400 km at a certain speed. Had the speed been 12 km/h more, the time taken for the journey would have been 1 hour 40 minutes less. Find the original speed of the car.
Chapter: [0.06] Solving (Simple) Problems (Based on Quadratic Equations)
Construct a triangle ABC in which base BC = 6 cm, AB = 5.5 cm and ∠ABC = 120°.
Construct a circle circumscribing the triangle ABC.
Draw a cyclic quadrilateral ABCD so that D is equidistant from B and C.
Chapter: [0.18] Constructions
The following distribution represents the height of 160 students of a school.
| Height (in cm) | No. of Students |
| 140 – 145 | 12 |
| 145 – 150 | 20 |
| 150 – 155 | 30 |
| 155 – 160 | 38 |
| 160 – 165 | 24 |
| 165 – 170 | 16 |
| 170 – 175 | 12 |
| 175 – 180 | 8 |
Draw an ogive for the given distribution taking 2 cm = 5 cm of height on one axis and 2 cm = 20 students on the other axis. Using the graph, determine:
- The median height.
- The interquartile range.
- The number of students whose height is above 172 cm.
Chapter: [0.06] Statistics
In triangle PQR, PQ = 24 cm, QR = –7 cm and ∠PQR = 90°. Find the radius of the inscribed circle.

Chapter: [0.17] Circles
Find the mode and the median of the following frequency distribution:
| x | 10 | 11 | 12 | 13 | 14 | 15 |
| f | 1 | 4 | 7 | 5 | 9 | 3 |
Chapter: [0.06] Statistics
The line through P(5, 3) intersects y-axis at Q.
(1) Write the slope of the line.
(2) Write the equation of the line.
(3) Find the coordinates of Q.
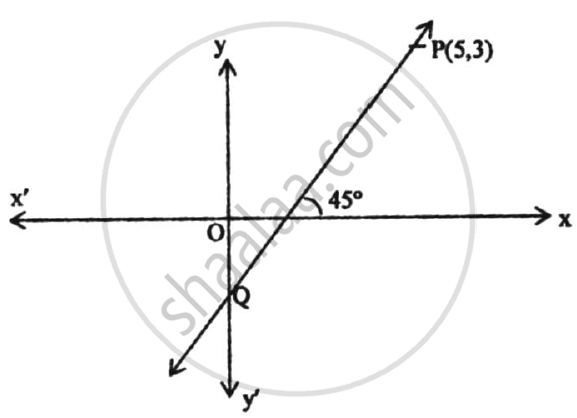
Chapter: [0.14] Co-ordinate Geometry Equation of a Line
Other Solutions
Submit Question Paper
Help us maintain new question papers on Shaalaa.com, so we can continue to help studentsonly jpg, png and pdf files
CISCE previous year question papers ICSE Class 10 Mathematics with solutions 2011 - 2012
Previous year Question paper for CISCE ICSE Class 10 Maths-2012 is solved by experts. Solved question papers gives you the chance to check yourself after your mock test.
By referring the question paper Solutions for Mathematics, you can scale your preparation level and work on your weak areas. It will also help the candidates in developing the time-management skills. Practice makes perfect, and there is no better way to practice than to attempt previous year question paper solutions of CISCE ICSE Class 10 .
How CISCE ICSE Class 10 Question Paper solutions Help Students ?
• Question paper solutions for Mathematics will helps students to prepare for exam.
• Question paper with answer will boost students confidence in exam time and also give you an idea About the important questions and topics to be prepared for the board exam.
• For finding solution of question papers no need to refer so multiple sources like textbook or guides.
