Advertisements
Advertisements
Question
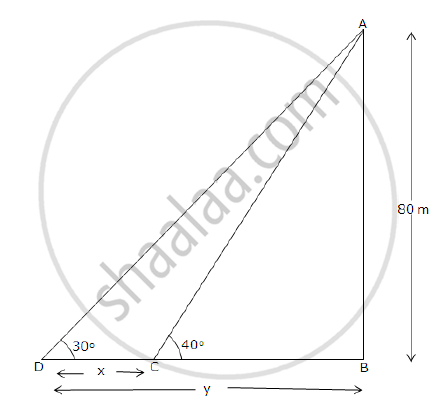
As observed from the top of an 80 m tall lighthouse, the angles of depression of two ships on the same side of the lighthouse of the horizontal line with its base are 30° and 40° respectively. Find the distance between the two ships. Give your answer correct to the nearest meter.
Solution
Let AB be the lighthouse and C and D be the two ships.
The angles of depression of the 2 ships are 30° and 40°
So, ∠ADB = 30°
∠ACB = 40°
Let the distance between the ships be CD = x m.
Also, Let BD = y m.
In ΔABC
`tan 40^@ = 80/(y - x)`
`=> y - x = 80/0.8390 = 95.352` ....(1)
Also from ΔABD
`tan 30^@ = 80/y`
`=> y = 80sqrt3 = 80 xx 1.732m` = 138.56 m
From (1) we get
`138.56 - x = 95.352`
`=> x = 138.56 - 95.352 cm = 43.208 m ~~ 43m`
APPEARS IN
RELATED QUESTIONS
Prove the following trigonometric identities.
(cosec θ − sec θ) (cot θ − tan θ) = (cosec θ + sec θ) ( sec θ cosec θ − 2)
Prove that:
`tanA/(1 - cotA) + cotA/(1 - tanA) = secA cosecA + 1`
If 4 cos2 A – 3 = 0, show that: cos 3 A = 4 cos3 A – 3 cos A
Prove the following identity :
(secA - cosA)(secA + cosA) = `sin^2A + tan^2A`
Prove the following identity :
`tanA - cotA = (1 - 2cos^2A)/(sinAcosA)`
If x = asecθ + btanθ and y = atanθ + bsecθ , prove that `x^2 - y^2 = a^2 - b^2`
A moving boat is observed from the top of a 150 m high cliff moving away from the cliff. The angle of depression of the boat changes from 60° to 45° in 2 minutes. Find the speed of the boat in m/min.
Prove that sin (90° - θ) cos (90° - θ) = tan θ. cos2θ.
If cosec θ + cot θ = p, then prove that cos θ = `(p^2 - 1)/(p^2 + 1)`
If sinθ = `11/61`, then find the value of cosθ using the trigonometric identity.
