Topics
Basic Concepts in Geometry
- Introduction to Basic Concepts in Geometry
- Concept of Points
- Concept of Line
- Concept of Plane
- Co-ordinates of Points and Distance
- Betweenness
- Concept of Line Segment
- Concept of Ray
- Conditional Statements and Converse
- Proofs
Parallel Line
- Introduction to Parallel Lines
- Pairs of Lines - Transversal of Parallel Lines
- Properties of Parallel Lines
- Interior Angle Theorem
- Corresponding Angle Theorem
- Alternate Angles Theorems
- Use of properties of parallel lines
- Test for Parallel Lines
- Interior Angles Test
- Concept of Angle
- Corresponding Angles Test
- Corollary of Parallel Lines
Triangles
- Concept of Triangles
- Remote Interior Angles of a Triangle Theorem
- Exterior Angle of a Triangle and Its Property
- Congruence of Triangles
- Isosceles Triangles Theorem
- Converse of Isosceles Triangle Theorem
- Corollary of a Triangle
- Property of 30°- 60°- 90° Triangle Theorem
- Property of 45°- 45°- 90° Triangle Theorem
- Median of a Triangle
- Property of Median Drawn on the Hypotenuse of Right Triangle
- Perpendicular Bisector Theorem
- Angle Bisector Theorem
- Properties of inequalities of sides and angles of a triangle
- Similar Triangles
- Similarity of Triangles
Constructions of Triangles
- Perpendicular Bisector Theorem
- Construction of Triangles
- To Construct a Triangle When Its Base, an Angle Adjacent to the Base, and the Sum of the Lengths of Remaining Sides is Given.
- To Construct a Triangle When Its Base, Angle Adjacent to the Base and Difference Between the Remaining Sides is Given.
- To Construct a Triangle, If Its Perimeter, Base and the Angles Which Include the Base Are Given.
Quadrilaterals
- Concept of Quadrilaterals
- Properties of a Parallelogram
- Properties of Rhombus
- Properties of a Square
- Properties of Rectangle
- Properties of Trapezium
- Property: The Opposite Sides of a Parallelogram Are of Equal Length.
- Property: The Opposite Angles of a Parallelogram Are of Equal Measure.
- Property: The diagonals of a parallelogram bisect each other. (at the point of their intersection)
- Property: The adjacent angles in a parallelogram are supplementary.
- Tests for Parallelogram
- Theorem : If Each Pair of Opposite Sides of a Quadrilateral is Equal, Then It is a Parallelogram.
- Theorem: If in a Quadrilateral, Each Pair of Opposite Angles is Equal, Then It is a Parallelogram.
- Theorem : If the Diagonals of a Quadrilateral Bisect Each Other, Then It is a Parallelogram
- Theorem: If One Pair of Opposite Sides of a Quadrilateral Are Equal and Parallel, It is a Parallelogram.
- Property: The Diagonals of a Rectangle Are of Equal Length.
- Property: Diagonals of a Square Are Congruent.
- Property: The diagonals of a square are perpendicular bisectors of each other.
- Property: Diagonals of a Square Bisect Its Opposite Angles.
- Property: The diagonals of a rhombus are perpendicular bisectors of one another.
- Property: Diagonals of a Rhombus Bisect Its Opposite Angles.
- Properties of Isosceles Trapezium
- Theorem of Midpoints of Two Sides of a Triangle
- Converse of Mid-point Theorem
Circle
- Concept of Circle
- Properties of Chord
- Theorem: a Perpendicular Drawn from the Centre of a Circle on Its Chord Bisects the Chord.
- Theorem : The Segment Joining the Centre of a Circle and the Midpoint of Its Chord is Perpendicular to the Chord.
- Relation Between Congruent Chords of a Circle and Their Distances from the Centre
- Properties of Congruent Chords
- Theorem: Equal chords of a circle are equidistant from the centre.
- Theorem : The Chords of a Circle Which Are Equidistant from the Centre Are Equal.
- Incircle of a Triangle
- Construction of the Incircle of a Triangle.
- Circumcentre of a Triangle
- Construction of the Circumcircle of a Triangle
Co-ordinate Geometry
- Coordinate Geometry
- The Co-ordinates of a Point in a Plane
- Co-ordinates of Points on the Axes
- Plotting a Point in the Plane If Its Coordinates Are Given.
- Equations of Lines Parallel to the X-axis and Y-axis
- Graphs of Linear Equations
- The Graph of a Linear Equation in the General Form
Trigonometry
- Trigonometry
- Terms Related to Right Angled Triangle
- Trigonometric Ratios and Its Reciprocal
- Relation Among Trigonometric Ratios
- Trigonometric Ratios of 30° and 60° Angles
- Trigonometric Table
- Important Equation in Trigonometry
Surface area and volume
- Definition
- Properties and Representation of a Ray
Definition
Rays: A ray is a portion of a line that begins at a fixed point, called its origin, and extends infinitely in one direction.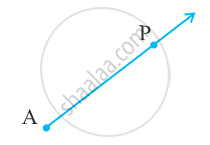
Properties and Representation of a Ray
- A ray has only one end (fixed) point, which is also known as its initial point.
- A ray extends indefinitely in only one direction.
- An unlimited number of rays can be drawn with the same initial point.
- A ray is a part of a line
- A ray starting from point P and passing through Q is denoted by `vec(PQ)`.

If you would like to contribute notes or other learning material, please submit them using the button below.
Shaalaa.com | Concept of Ray
to track your progress