Addition and Subtraction of Vectors - Graphical Method

The resultant of two vectors `vecA` and `vecB` is `vecC`. If the magnitude of `vecB` is doubled, the new resultant vector becomes perpendicular to `vecA`, then the magnitude of `vecC` is

If `vecA` and `vecB` is two vectors satisfying the relation `vecA.vecB = [vecA xx vecB]`. Then the value of `[vecA - vecB]` will be ______.

Establish the following vector inequalities geometrically or otherwise:

1. | a+b | ≤ |a| + |b|
2. |a + b| ≥ | |a| - |b| |
3. |a - b| ≤ |a| + |b|
4. |a-b| ≥ | |a| - |b| |

When does the equality sign above apply?

Given a + b + c + d = 0, state whether the following statement is correct or incorrect: 

The magnitude of (a + c) equals the magnitude of (b + d).

Given below in column I are the relations between vectors a, b and c and in column II are the orientations of a, b and c in the XY plane. Match the relation in column I to correct orientations in column II.

Column I			Column II
(a)	a + b = c	(i)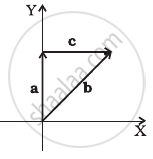
(b)	a – c = b	(ii)
(c)	b – a = c	(iii)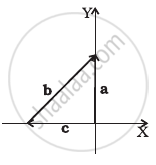
(d)	a + b + c = 0	(iv)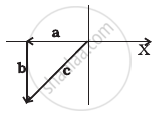

For two vectors A and B, A + B = A − B is always true when

1. |A| = |B| ≠ 0
2. A ⊥ B
3. |A| = |B| ≠ 0 and A and B are parallel or anti parallel
4. When either |A| or |B| is zero

Given a + b + c + d = 0, state whether the following statement is correct or incorrect:

b + c must lie in the plane of a and d if a and d are not collinear, and in the line of a and d, if they are collinear.

If `vec"A" = (2hat"i"+3hat"j"- hat"k")`m and `vec"B" = (hat"i" + 2hat"j"+ 2hat"k")` m. The magnitude of component of vector `vec"A"` along vector `vec"B"` will be ______ m.