Advertisements
Advertisements
प्रश्न
Derive Laplace’s law for spherical membrane of bubble due to surface tension.
Derive Laplace’s law for a spherical membrane.
उत्तर १
Consider a spherical liquid drop and let the outside pressure be Po and inside pressure be Pi, such that the excess pressure is Pi − Po
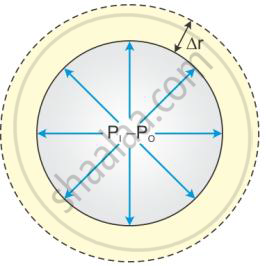
Let the radius of the drop increase from r to Δr, where Δr is very small, so that the pressure inside the drop remains almost constant.
Initial surface area (A1) = 4Πr2
Final surface area (A2) = 4Π(r + Δr)2
= 4π(r2 + 2rΔr + Δr2)
= 4Πr2 + 8ΠrΔr + 4ΠΔr2
As Δr is very small, Δr2 is neglected (i.e. 4πΔr2≅0)
Increase in surface area (dA) =A2 - A1= 4Πr2 + 8ΠrΔr - 4Πr2
Increase in surface area (dA) =8ΠΔr
Work done to increase the surface area by 8ΠrΔr is extra energy.
∴ dW = TdA
∴ dW = T*8πrΔr .......(Equ.1)
This work done is equal to the product of the force and the distance Δr.
dF=(P1 - P0)4πr2
The increase in the radius of the bubble is Δr.
dW = dFΔr = (P1 - P0)4Πr2*Δr ..........(Equ.2)
Comparing Equations 1 and 2, we get
(P1 - P0)4πr2*Δr = T*8πrΔr
∴`(P_1 - P_0) = (2T)/R`
This is called Laplace’s law of spherical membrane.
उत्तर २
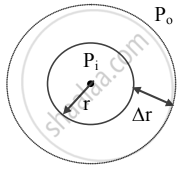
- The free surface of drops or bubbles is spherical in shape.
Let,
Pi = inside pressure of a drop or air bubble
Po = outside pressure of the bubble
r = radius of drop or bubble. - As drop is spherical, Pi > Po
∴ excess pressure inside drop = Pi − Po - Let the radius of drop increase from r to r + ∆r so that inside pressure remains constant.
- Initial area of drop A1 = 4πr2 ,
Final surface area of drop A2 = 4π (r + ∆r)2
Increase in surface area,
∆A = A2 − A1 = 4π[(r + ∆r)2 − r2]
= 4π[r2 + 2r∆r + ∆r2 − r2]
= 8πr∆r + 4π∆r2 - As ∆r is very small, the term containing ∆r2 can be neglected.
∴ dA = 8πr∆r - Work is done by a force of surface tension,
dW = TdA = (8πr∆r)T ….(1)
This work done is also equal to the product of the force F which causes an increase in the area of the bubble and the displacement Δr which is the increase in the radius of the bubble.
∴ dW = FΔr
The excess force is given by,
(Excess pressure) × (Surface area)
∴ F = (Pi – Po) × 4πr2
∴ dF = (Pi – Po)A
dW = F∆r = (Pi − Po) A∆r
From equation (1),
(Pi − Po) A∆r = (8πr∆r) T
∴ Pi − Po = `(8pirDeltarT)/(4pir^2Deltar)` ........(∵ A = 4πr2)
∴ Pi − Po = `(2T)/r` ….(2)
Equation (2) represents Laplace’s law of spherical membrane.
APPEARS IN
संबंधित प्रश्न
A raindrop of diameter 4 mm is about to fall on the ground. Calculate the pressure inside the raindrop. [Surface tension of water T = 0.072 N/m, atmospheric pressure = 1.013 x 105 N/m2 ]
Define the angle of contact.
Water rises to a height 3.2 cm in a glass capillary tube. Find the height to which the same water will rise in another glass capillary having half area of cross section.
Explain why Water with detergent dissolved in it should have small angles of contact.
Explain why A drop of liquid under no external forces is always spherical in shape
Mercury has an angle of contact equal to 140° with soda lime glass. A narrow tube of radius 1.00 mm made of this glass is dipped in a trough containing mercury. By what amount does the mercury dip down in the tube relative to the liquid surface outside? Surface tension of mercury at the temperature of the experiment is 0.465 N m–1. Density of mercury = 13.6 × 103 kg m–3
Show that the surface tension of a liquid is numerically equal to the surface energy per unit
area.
A big drop of radius R is formed from 1000 droplets of water. The radius of a droplet will be _______
A) 10 R
B) R/10
C) R/100
D) R/1000
State any two characteristics of the angle of contact
The free surface of a liquid resting in an inertial frame is horizontal. Does the normal to the free surface pass through the centre of the earth? Think separately if the liquid is (a) at the equator (b) at a pole (c) somewhere else.
The contact angle between water and glass is 0°. When water is poured in a glass to the maximum of its capacity, the water surface is convex upward. The angle of contact in such a situation is more than 90°. Explain.
A uniform vertical tube of circular cross section contains a liquid. The contact angle is 90°. Consider a diameter of the tube lying in the surface of the liquid. The surface to the right of this diameter pulls the surface on the left of it. What keeps the surface on the left in equilibrium?
If a mosquito is dipped into water and released, it is not able to fly till it is dry again. Explain
The force of surface tension acts tangentially to the surface whereas the force due to air pressure acts perpendicularly on the surface. How is then the force due to excess pressure inside a bubble balanced by the force due to the surface tension?
Frictional force between solids operates even when they do not move with respect to each other. Do we have viscous force acting between two layers even if there is no relative motion?
When water droplets merge to form a bigger drop
If two soap bubbles of different radii are connected by a tube,
Which of the following graphs may represent the relation between the capillary rise hand the radius r of the capillary?
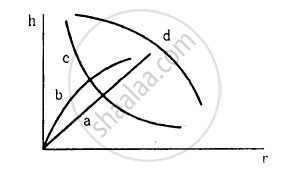
Water rises in a vertical capillary tube up to a length of 10 cm. If the tube is inclined at 45°, the length of water risen in the tube will be
A capillary tube of radius 0.50 mm is dipped vertically in a pot of water. Find the difference between the pressure of the water in the tube 5.0 cm below the surface and the atmospheric pressure. Surface tension of water = 0.075 N m−1.
A drop of mercury of radius 2 mm is split into 8 identical droplets. Find the increase in surface energy. Surface tension of mercury = 0.465 J m−2.
Find the force exerted by the water on a 2 m2 plane surface of a large stone placed at the bottom of a sea 500 m deep. Does the force depend on the orientation of the surface?
A metal piece of mass 160 g lies in equilibrium inside a glass of water. The piece touches the bottom of the glass at a small number of points. If the density of the metal is 8000 kg/m3, find the normal force exerted by the bottom of the glass on the metal piece.

A cube of ice floats partly in water and partly in K.oil (in the following figure). Find the ratio of the volume of ice immersed in water to that in K.oil. Specific gravity of K.oil is 0.8 and that of ice is 0.9.

A cubical block of wood weighing 200 g has a lead piece fastened underneath. Find the mass of the lead piece which will just allow the block to float in water. Specific gravity of wood is 0.8 and that of lead is 11.3.
Calculate the rise of water inside a clean glass capillary tube of radius 0.1 mm, when immersed in water of surface tension 7 × 10-2 N/m. The angle of contact between water and glass is zero, the density of water = 1000 kg/m3, g = 9.8 m/s2.
Insect moves over the surface of water because of ______.
Define surface tension
Water rises to a height of 20 mm in a capillary tube. If the radius made 1/3rd of its previous value, to what height will the water now rise in the tube?
Two soap bubbles have a radius in the ratio of 2:3. Compare the works done in blowing these bubbles.
A u-tube is made up of capillaries of bore 1 mm and 2 mm respectively. The tube is held vertically and partially filled with a liquid of surface tension 49 dyne/cm and zero angles of contact. Calculate the density of the liquid, if the difference in the levels of the meniscus is 1.25 cm. take g = 980 cm/s2
The property of _______ of a liquid surface enables the water droplets to move upward in plants.
Numerical Problem.
A stone weighs 500 N. Calculate the pressure exerted by it if it makes contact with a surface of area 25 cm2.
How does the friction arise between the surfaces of two bodies in relative motion?
Distinguish between cohesive and adhesive forces.
Obtain an expression for the surface tension of a liquid by the capillary rise method.
Why coffee runs up into a sugar lump (a small cube of sugar) when one corner of the sugar lump is held in the liquid?
Two spherical rain drops reach the surface of the earth with terminal velocities having ratio 16 : 9. The ratio of their surface area is ______.
Water rises upto a height h in a capillary tube on the surface of the earth. The value of h will increase, if the experimental setup is kept in [g = acceleration due to gravity]
If the surface tension of a soap solution is 3 × 10-2 N/m then the work done in forming a soap film of 20 cm × 5 cm will be ______.
The excess of pressure, due to surface tension, on a spherical liquid drop of radius 'R' is proportional to ______.
Under isothermal conditions, two soap bubbles of radii 'r1' and 'r2' coalesce to form a big drop. The radius of the big drop is ______.
Soap solution is used for cleaning dirty clothes because ______.
Is surface tension a vector?
The sap in trees, which consists mainly of water in summer, rises in a system of capillaries of radius r = 2.5 × 10–5 m. The surface tension of sap is T = 7.28 × 10–2 Nm–1 and the angle of contact is 0°. Does surface tension alone account for the supply of water to the top of all trees?
Two mercury droplets of radii 0.1 cm. and 0.2 cm. collapse into one single drop. What amount of energy is released? The surface tension of mercury T = 435.5 × 10–3 Nm–1.
This model of the atmosphere works for relatively small distances. Identify the underlying assumption that limits the model.
Eight droplets of water each of radius 0.2 mm coalesce into a single drop. Find the decrease in the surface area.
A soap bubble of radius 3 cm is formed inside another soap bubble of radius 6 cm. The radius of an equivalent soap bubble which has the same excess pressure as inside the smaller bubble with respect to the atmospheric pressure is ______ cm.
A liquid flows out drop by drop from a vessel through a vertical tube with an internal diameter of 2 mm, then the total number of drops that flows out during 10 grams of the liquid flow out ______. [Assume that the diameter of the neck of a drop at the moment it breaks away is equal to the internal diameter of tube and surface tension is 0.02 N/m].
A soap film of surface tension 3 × 10-2 formed in a rectangular frame can support a straw as shown in Fig. If g = 10 ms-12, the mass of the straw is ______.

When one end of the capillary is dipped in water, the height of water column is 'h'. The upward force of 105 dyne due to surface tension is balanced by the force due to the weight of water column. The inner circumference of capillary is ______.
(Surface tension of water = 7 × 10-2 N/m)
