Advertisements
Advertisements
प्रश्न
Find the area of a triangle with vertices at the point given in the following:
(−2, −3), (3, 2), (−1, −8)
उत्तर
Area of the triangle passing through the vertices `(x_1,y_1),(x_2,y_2),(x_3,y_3)`,
`Delta = 1/2 abs ((x_1,y_1,1),(x_2,y_2,1),(x_3,y_3,1))`
`Delta = 1/2 abs ((-2,-3,1),(3,2,1),(-1,-8,1))`
`= 1/2 [-2 ( 2 + 8) + 3 (3 + 1) + (-24 + 2)]`
`= 1/2 [-2 (10) + 3 (4) + (- 22)]`
`= 1/2 [-20 + 12 - 22]`
`= - 30/2`
= - 15
∴ Area = 15 square units. (As area > 0)
APPEARS IN
संबंधित प्रश्न
Prove that the area of a triangle with vertices (t, t −2), (t + 2, t + 2) and (t + 3, t) is independent of t.
The perimeter of a right triangle is 60 cm. Its hypotenuse is 25 cm. Find the area of the triangle.
If A(−4, 8), B(−3, −4), C(0, −5) and D(5, 6) are the vertices of a quadrilateral ABCD, find its area.
If the points A(−1, −4), B(b, c) and C(5, −1) are collinear and 2b + c = 4, find the values of b and c.
Prove that the points (2, – 2), (–3, 8) and (–1, 4) are collinear
Prove that the points (a, b + c), (b, c + a) and (c, a + b) are collinear
In each of the following find the value of 'k', for which the points are collinear.
(8, 1), (k, -4), (2, -5)
Find a relation between x and y if the points (x, y), (1, 2) and (7, 0) are collinear.
Find the centre of a circle passing through the points (6, − 6), (3, − 7) and (3, 3).
The two opposite vertices of a square are (− 1, 2) and (3, 2). Find the coordinates of the other two vertices.
Find values of k if area of triangle is 4 square units and vertices are (k, 0), (4, 0), (0, 2)
Find the area of a triangle whose vertices are
(a, c + a), (a, c) and (−a, c − a)
Find the area of the quadrilaterals, the coordinates of whose vertices are
(−3, 2), (5, 4), (7, −6) and (−5, −4)
Find the area of the quadrilaterals, the coordinates of whose vertices are
(1, 2), (6, 2), (5, 3) and (3, 4)
Prove that the points (2,3), (-4, -6) and (1, 3/2) do not form a triangle.
For what value of a point (a, 1), (1, -1) and (11, 4) are collinear?
Prove that the points (a, b), (a1, b1) and (a −a1, b −b1) are collinear if ab1 = a1b.
If G be the centroid of a triangle ABC and P be any other point in the plane, prove that PA2+ PB2 + PC2 = GA2 + GB2 + GC2 + 3GP2.
Find the area of a triangle two sides of which are 18 cm and 10 cm and the perimeter is 42cm ?
prove that the points A (7, 10), B(-2, 5) and C(3, -4) are the vertices of an isosceles right triangle.
Show that the points A (3,1) , B (0,-2) , C(1,1) and D (4,4) are the vertices of parallelogram ABCD.
Find the area of ΔABC whose vertices are:
A(10,-6) , B (2,5) and C(-1,-3)
Show that the following points are collinear:
A(-5,1), B(5, 5) and C(10, 7)
Find a relation between x and y, if the points A(2, 1), B(x, y) and C(7,5) are collinear.
Prove that the points A (a,0), B( 0,b) and C (1,1) are collinear, if `( 1/a+1/b) =1`.
Find the value(s) of p for which the points (3p + 1, p), (p + 2, p – 5) and (p + 1, –p) are collinear ?
The coordinates of the point P dividing the line segment joining the points A (1, 3) and B (4, 6) in the ratio 2 : 1 are:
Find the value(s) of k so that the quadratic equation x2 − 4kx + k = 0 has equal roots.
Find the area of the following triangle:

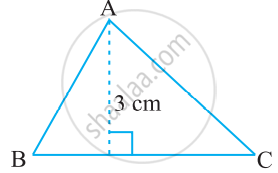
Find BC, if the area of the triangle ABC is 36 cm2 and the height AD is 3 cm.
A field is in the shape of a right angled triangle whose base is 25 m and height 20 m. Find the cost of levelling the field at the rate of ₹ 45 per sq.m2
If the points (2, -3), (k, -1), and (0, 4) are collinear, then find the value of 4k.
The area of a triangle with vertices (a, b + c), (b, c + a) and (c, a + b) is ______.
The points (0, 5), (0, –9) and (3, 6) are collinear.
Using determinants, find the area of ΔPQR with vertices P(3, 1), Q(9, 3) and R(5, 7). Also, find the equation of line PQ using determinants.
