Advertisements
Advertisements
प्रश्न
If \[3f\left( x \right) + 5f\left( \frac{1}{x} \right) = \frac{1}{x} - 3\] for all non-zero x, then f(x) =
विकल्प
(a) \[\frac{1}{14}\left( \frac{3}{x} + 5x - 6 \right)\]
(b) \[\frac{1}{14}\left( - \frac{3}{x} + 5x - 6 \right)\]
(c) \[\frac{1}{14}\left( - \frac{3}{x} + 5x + 6 \right)\]
(d) None of these
उत्तर
(d) None of these
\[3f\left( x \right) + 5f\left( \frac{1}{x} \right) = \frac{1}{x} - 3\]
\[\text{ Multiplying (1) by } 3: \]
\[15 f\left( \frac{1}{x} \right) + 9 f(x) = \frac{3}{x} - 9 . . . . . (2)\]
\[\text{ Replacing x by} \frac{1}{x}\text{ in } (1): \]
\[3 f\left( \frac{1}{x} \right) + 5 f(x) = x - 3 \]
\[\text{ Multiplying by } 5: \]
\[15 f\left( \frac{1}{x} \right) + 25 f(x) = 5x - 15 . . . . (3)\]
\[\text{ Solving (2) and (3) } : \]
\[ - 16 f(x) = \frac{3}{x} - 5x + 6\]
\[ \Rightarrow f(x) = \frac{1}{16}\left( - \frac{3}{x} + 5x - 6 \right)\]
Notes
Disclaimer: The question in the book has some error, so, none of the options are matching with the solution. The solution is created according to the question given in the book.
APPEARS IN
संबंधित प्रश्न
Let f be the subset of Z × Z defined by f = {(ab, a + b): a, b ∈ Z}. Is f a function from Z to Z: justify your answer.
Let A = {9, 10, 11, 12, 13} and let f: A → N be defined by f(n) = the highest prime factor of n. Find the range of f.
Let X = {1, 2, 3, 4} and Y = {1, 5, 9, 11, 15, 16}
Determine which of the set are functions from X to Y.
(c) f3 = {(1, 5), (2, 9), (3, 1), (4, 5), (2, 11)}
Let f and g be two real functions defined by \[f\left( x \right) = \sqrt{x + 1}\] and \[g\left( x \right) = \sqrt{9 - x^2}\] . Then, describe function:
(ii) g − f
Write the range of the function f(x) = cos [x], where \[\frac{- \pi}{2} < x < \frac{\pi}{2}\] .
Write the domain and range of the function \[f\left( x \right) = \frac{x - 2}{2 - x}\] .
If \[f\left( x \right) = \log \left( \frac{1 + x}{1 - x} \right) \text{ and} g\left( x \right) = \frac{3x + x^3}{1 + 3 x^2}\] , then f(g(x)) is equal to
If A = {1, 2, 3} and B = {x, y}, then the number of functions that can be defined from A into B is
Let f : R → R be defined by f(x) = 2x + |x|. Then f(2x) + f(−x) − f(x) =
If f : R → R and g : R → R are defined by f(x) = 2x + 3 and g(x) = x2 + 7, then the values of x such that g(f(x)) = 8 are
The domain of the function \[f\left( x \right) = \sqrt{5 \left| x \right| - x^2 - 6}\] is
The range of the function \[f\left( x \right) = \frac{x}{\left| x \right|}\] is
If f(x) = 3x + a and f(1) = 7 find a and f(4).
If f(x) = `{(x^2 + 3"," x ≤ 2),(5x + 7"," x > 2):},` then find f(0)
Check if the following relation is a function.
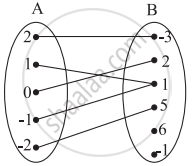
Check if the following relation is a function.
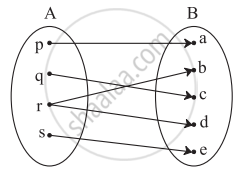
Check if the relation given by the equation represents y as function of x:
2x + 3y = 12
If f(m) = m2 − 3m + 1, find f(−3)
If f(m) = m2 − 3m + 1, find `(("f"(2 + "h") - "f"(2))/"h"), "h" ≠ 0`
Find the domain and range of the following function.
f(x) = `sqrt((x - 2)(5 - x)`
Find the domain and range of the following function.
f(x) = `sqrt((x - 3)/(7 - x))`
Show that if f : A → B and g : B → C are one-one, then g ° f is also one-one
Write the following expression as a single logarithm.
ln (x + 2) + ln (x − 2) − 3 ln (x + 5)
Solve for x.
log2 + log(x + 3) – log(3x – 5) = log3
Solve for x.
2 log10 x = `1 + log_10 (x + 11/10)`
Solve for x.
log2 x + log4 x + log16 x = `21/4`
Answer the following:
Find whether the following function is one-one
f : R → R defined by f(x) = x2 + 5
Answer the following:
Simplify, log (log x4) – log (log x)
Answer the following:
If a2 = b3 = c4 = d5, show that loga bcd = `47/30`
Answer the following:
Find the range of the following function.
f(x) = |x – 5|
A graph representing the function f(x) is given in it is clear that f(9) = 2
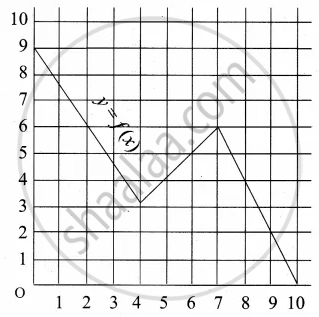
Find the following values of the function
(a) f(0)
(b) f(7)
(c) f(2)
(d) f(10)
A graph representing the function f(x) is given in it is clear that f(9) = 2
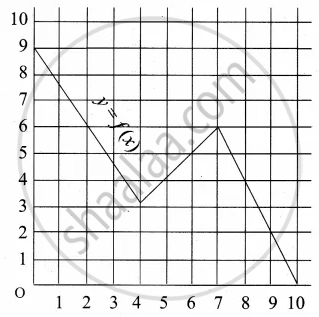
Describe the following Range
A graph representing the function f(x) is given in it is clear that f(9) = 2

What is the image of 6 under f?
Mapping f: R → R which is defined as f(x) = sin x, x ∈ R will be ______
Domain of function f(x) = cos–1 6x is ______.
Find the domain of the following function.
f(x) = [x] + x
