Advertisements
Advertisements
प्रश्न
If A = 600 and B = 300, verify that:
(i) sin (A + B) = sin A cos B + cos A sin B
उत्तर
A = 600 and B = 300
Now, A + B = 600 + 300 = 900
Also, A – B = 600 – 300 = 300
(i) sin (A + B) = sin 900 = 1
sin A cos B + cos A sin B = sin 600 cos 300 + cos 600 sin 300
=`(sqrt(3)/2xx sqrt(3)/2 + 1/2xx1/2) = (3/4+1/4)=1`
∴ sin (A + B) = sin A cos B + cos A sin B
APPEARS IN
संबंधित प्रश्न
If Sin (A + B) = 1 and cos (A – B) = 1, 0° < A + B ≤ 90° A ≥ B. Find A & B
If sin θ = ` (a^2 - b^2)/(a^2+b^2)`find all the values of all T-ratios of θ .
If A = 300 , verify that:
(i) sin 2A = `(2 tan A)/(1+tan^2A)`
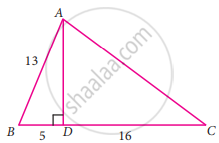
From the following figure, find the values of
(i) cos A
(ii) cosec A
(iii) tan2A - sec2A
(iv) sin C
(v) sec C
(vi) cot2 C - ` 1 / sin^2 "c"`

From the following figure, find:
(i) y
(ii) sin x°
(iii) (sec x° - tan x°) (sec x° + tan x°)

In the given figure, triangle ABC is right-angled at B. D is the foot of the perpendicular from B to AC. Given that BC = 3 cm and AB = 4 cm.
find :
- tan ∠DBC
- sin ∠DBA

In rectangle ABCD, diagonal BD = 26 cm and cotangent of angle ABD = 1.5. Find the area and the perimeter of the rectangle ABCD.
In the given figure, PQR is a triangle, in which QS ⊥ PR, QS = 3 cm, PS = 4 cm and QR = 12 cm, find the value of: 4sin2R - `(1)/("tan"^2"P")`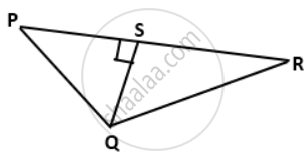
In the given figure, AD is the median on BC from A. If AD = 8 cm and BC = 12 cm, find the value of cos y
From the given figure, find the values of cosec C