Advertisements
Advertisements
प्रश्न
If the angles of a triangle are 30°, 60°, and 90°, then shown that the side opposite to 30° is half of the hypotenuse, and the side opposite to 60° is `sqrt(3)/2` times of the hypotenuse.
उत्तर
Given : In ΔCAB, m∠A=90°, m∠B = 60°, M∠C=30°
To prove : i AB = `1/2`BC ii. AC = `sqrt(3)/2 BC`
Construction: Take a point 'D' on ray BA such that AB = AD. join point C to point D.
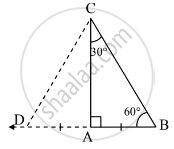
Proof: In ΔCBD,
AD= AB ....[By construction]
∴ A is the midpoint of seg BD ....(i)
Also, m∠CAB = 90° ....[Given]
∴ seg CA ⊥ seg BD .....(ii)
∴ seg CA is the perpendicular bisector of seg BD ....[From(i) and (ii)]
∴ CD = CB ...........[By perpendicular bisector theorem]
∴ ΔCDB is an isosceles triangle
∴ ∠CDB ≅ ∠CBD .....(iii)[By isosceles triangle theorem]
But,∠CBD = 60° ....(iv) [Given]
∴ ∠CDB = 60° ....[from (iii) and (iv)]
∴ ∠BCD = 60° .....[Remaining angle of a triangle ]
∴ ΔCDB is an equilateral triangle ....[All angle are 60°]
∴ BD = BC = CD ....(vi)[Sides of equilateral triabgle ]
AB = `1/2` BD .....(vi) [By construction]
AB = `1/2` BC . ...(vii) [ From (v) and (vi)]
In ΔCAB,
∠CAB = 90° ....[Given]
∴ BC2 = AC2+AB2 ............[ By pythagoras theorem]
∴` BC^2 = AC^2 + (1/2 BC)^2` ...[From (vii)]
∴`BC^2 = AC^2 +1/4 BC^2`
∴ `AC^2 = BC^2 -1/4 BC^2`
∴ `Ac^2 = (4BC^2-BC^32)/4`
∴ `AC^2 = (3BC^2)/4`
∴ `AC = sqrt(3)/2 BC` ...[ Taking square root on both sides]
APPEARS IN
संबंधित प्रश्न
A ladder leaning against a wall makes an angle of 60° with the horizontal. If the foot of the ladder is 2.5 m away from the wall, find the length of the ladder
D and E are points on the sides CA and CB respectively of a triangle ABC right angled at C. Prove that AE2 + BD2 = AB2 + DE2
In an equilateral triangle, prove that three times the square of one side is equal to four times the square of one of its altitudes.
A tree is broken at a height of 5 m from the ground and its top touches the ground at a distance of 12 m from the base of the tree. Find the original height of the tree.
Which of the following can be the sides of a right triangle?
1.5 cm, 2 cm, 2.5 cm
In the case of right-angled triangles, identify the right angles.
Find the perimeter of the rectangle whose length is 40 cm and a diagonal is 41 cm.
A ladder 13 m long rests against a vertical wall. If the foot of the ladder is 5 m from the foot of the wall, find the distance of the other end of the ladder from the ground.
In triangle ABC, given below, AB = 8 cm, BC = 6 cm and AC = 3 cm. Calculate the length of OC.

Prove that `(sin θ + cosec θ)^2 + (cos θ + sec θ)^2 = 7 + tan^2 θ + cot^2 θ`.
In the given figure, angle BAC = 90°, AC = 400 m, and AB = 300 m. Find the length of BC.

In a triangle ABC, AC > AB, D is the midpoint BC, and AE ⊥ BC. Prove that: AB2 = AD2 - BC x CE + `(1)/(4)"BC"^2`
In a right angled triangle, the hypotenuse is the greatest side
An isosceles triangle has equal sides each 13 cm and a base 24 cm in length. Find its height
Find the distance between the helicopter and the ship
In triangle ABC, line I, is a perpendicular bisector of BC.
If BC = 12 cm, SM = 8 cm, find CS
Choose the correct alternative:
If length of sides of a triangle are a, b, c and a2 + b2 = c2, then which type of triangle it is?
A flag pole 18 m high casts a shadow 9.6 m long. Find the distance of the top of the pole from the far end of the shadow.
Lengths of sides of a triangle are 3 cm, 4 cm and 5 cm. The triangle is ______.
Jayanti takes shortest route to her home by walking diagonally across a rectangular park. The park measures 60 metres × 80 metres. How much shorter is the route across the park than the route around its edges?
