Advertisements
Advertisements
प्रश्न
In ΔABC, BC produced to D, such that, AC = CD; ∠BAD = 125° and ∠ACD = 105°. Show that BC > CD.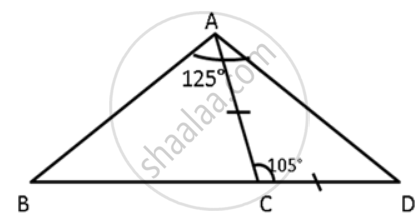
उत्तर
In ΔACD,
AC = CD ....(Given)
∠CDA = ∠DAC ....(ΔACD is isosceles triangle.)
Let ∠CDA = ∠DAC = x°
∠CDA + ∠DAC + ∠ACD = 180°
x° + x° + 105° = 180°
2x° + 180° - 105°
2x° = 75°
x = `(75°)/(2)`
x = 37.5°
∠C = ∠DAC = x° = 37.5° ......(i)
∠DAC =∠AC + ∠BAC
125° - 37.5° = ∠BAC ....from (i)
125° - 37.5° = ∠BAC
87.5° = ∠BAC
Also, ∠BCA + ∠ACD = 180°
⇒ ∠BCA + 105° = 180°
⇒ ∠BCA = 75°
So, in ΔBAC,
∠ACB + ∠BAC + ∠C = 180°
⇒ 75° + 87.5° + ∠ABC = 180°
⇒ ∠ABC = 17.5°
As 87.5° > 17.5°
∠BAC > ∠ABC
⇒ BC > AC
⇒ BC > CD. ....(Since AC = CD)
APPEARS IN
संबंधित प्रश्न
Show that in a right angled triangle, the hypotenuse is the longest side.
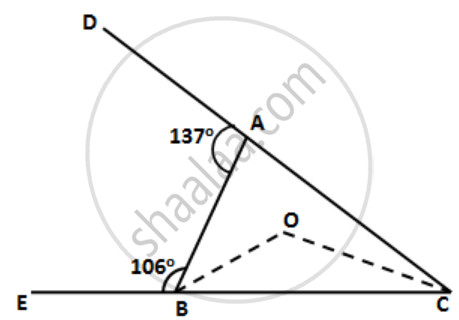
Arrange the sides of ∆BOC in descending order of their lengths. BO and CO are bisectors of angles ABC and ACB respectively.
Arrange the sides of the following triangles in an ascending order:
ΔABC, ∠A = 45°, ∠B = 65°.
In ΔABC, the exterior ∠PBC > exterior ∠QCB. Prove that AB > AC.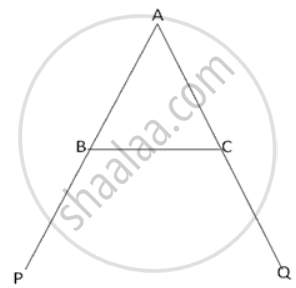
Prove that the hypotenuse is the longest side in a right-angled triangle.
D is a point on the side of the BC of ΔABC. Prove that the perimeter of ΔABC is greater than twice of AD.
In ABC, P, Q and R are points on AB, BC and AC respectively. Prove that AB + BC + AC > PQ + QR + PR.
In ΔPQR, PR > PQ and T is a point on PR such that PT = PQ. Prove that QR > TR.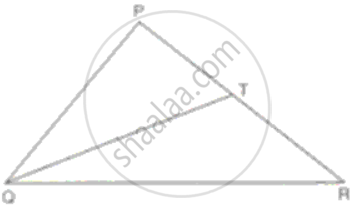
ABCD is a trapezium. Prove that:
CD + DA + AB + BC > 2AC.
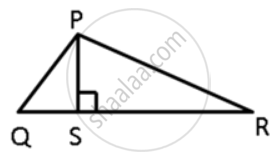
In ΔPQR, PS ⊥ QR ; prove that: PQ + PR > QR and PQ + QR >2PS.