Advertisements
Advertisements
प्रश्न
In right angled triangle ΔABC at B, ∠A = ∠C. Find the values of sin A sin B + cos A cos B
उत्तर
In Δle ABC ∠A + ∠B + ∠C = 180°
∠A + 90° + ∠A = 180°
2∠A = 90°
∠A = 45°
∴ ∠A = 45° ....(1)
Adding ∠A = 45° (1) in sin A sin B + cos A cos B
∠A = 45° sin 90^@ + cos 45^@ cos 90^@
`1/sqrt2 . 1 + 0`
`= 1/sqrt2 . 1 + 0`
`= 1/sqrt2`
APPEARS IN
संबंधित प्रश्न
In Fig below, Find tan P and cot R. Is tan P = cot R?

If `sin (A – B) = 1/2` and `cos (A + B) = 1/2`, `0^@` < A + `B <= 90^@`, A > B Find A and B.
In ∆PQR, right-angled at Q, PQ = 3 cm and PR = 6 cm. Determine ∠P and ∠R.
If sin θ ,` sqrt (3)/2` find the value of all T- ratios of θ .
If tan `theta = a/b`, show that `((a sin theta - b cos theta))/((a sin theta + bcos theta))= ((a^2-b^2))/(a^2+b^2)`
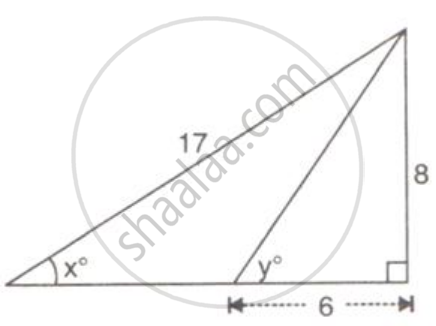
Use the given figure to find :
(i) sin xo
(ii) cos yo
(iii) 3 tan xo - 2 sin yo + 4 cos yo.
Given : 5 cos A - 12 sin A = 0; evaluate:
`(sin "A"+cos"A")/(2 cos"A"– sin"A")`
If sin A = `(sqrt3)/(2)` and cos B = `(sqrt3)/(2)` , find the value of : `(tan"A" – tan"B")/(1+tan"A" tan"B")`
In the given figure, PQR is a triangle, in which QS ⊥ PR, QS = 3 cm, PS = 4 cm and QR = 12 cm, find the value of: sin P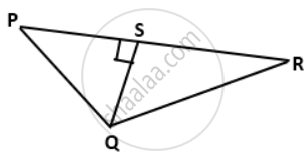
In the given figure, AD is the median on BC from A. If AD = 8 cm and BC = 12 cm, find the value of cos y
