Advertisements
Advertisements
प्रश्न
Which of the following relations are functions? Give reasons. If it is a function, determine its domain and range.
- {(2, 1), (5, 1), (8, 1), (11, 1), (14, 1), (17, 1)}
- {(2, 1), (4, 2), (6, 3), (8, 4), (10, 5), (12, 6), (14, 7)}
- {(1, 3), (1, 5), (2, 5)}
उत्तर
- Let R = {(2, 1), (5, 1), (8, 1), (11, 1), (14, 1), (17, 1)} This relation is a function because the first element of any two ordered pairs is not equal. Domain = {2, 6, 8, 11, 14, 17} and range = {1}
- Let R = {(2, 1), (4, 2), (6, 3), (8, 4), (10, 5), (12, 6), (14, 7)} It is a function because the first element of any two ordered pairs is not equal. Hence, domain = {2, 4, 6, 8, 10, 12, 14}, range = {1, 2, 3, 4, 5, 6, 7}.
- It is not a function because (1, 3), (1,5) have the same first element.
APPEARS IN
संबंधित प्रश्न
Find the domain of the function f(x) = `(x^2 + 2x + 1)/(x^2 - 8x + 12)`
Let A = {−2, −1, 0, 1, 2} and f : A → Z be a function defined by f(x) = x2 − 2x − 3. Find:
(a) range of f, i.e. f(A).
f, g, h are three function defined from R to R as follow:
(ii) g(x) = sin x
Find the range of function.
et A = (12, 13, 14, 15, 16, 17) and f : A → Z be a function given by
f(x) = highest prime factor of x.
Find range of f.
Let A = [p, q, r, s] and B = [1, 2, 3]. Which of the following relations from A to B is not a function?
If \[f\left( x \right) = \begin{cases}x^2 , & \text{ when } x < 0 \\ x, & \text{ when } 0 \leq x < 1 \\ \frac{1}{x}, & \text{ when } x \geq 1\end{cases}\]
find: (a) f(1/2), (b) f(−2), (c) f(1), (d)
Let f and g be two real functions defined by \[f\left( x \right) = \sqrt{x + 1}\] and \[g\left( x \right) = \sqrt{9 - x^2}\] . Then, describe function:
(iv) \[\frac{f}{g}\]
Let f and g be two real functions defined by \[f\left( x \right) = \sqrt{x + 1}\] and \[g\left( x \right) = \sqrt{9 - x^2}\] . Then, describe function:
(viii) \[\frac{5}{8}\]
Write the domain and range of function f(x) given by
Write the domain and range of function f(x) given by \[f\left( x \right) = \sqrt{\left[ x \right] - x}\] .
If f : R → R and g : R → R are defined by f(x) = 2x + 3 and g(x) = x2 + 7, then the values of x such that g(f(x)) = 8 are
f is a real valued function given by \[f\left( x \right) = 27 x^3 + \frac{1}{x^3}\] and α, β are roots of \[3x + \frac{1}{x} = 12\] . Then,
Check if the following relation is function:
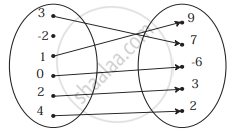
Check if the relation given by the equation represents y as function of x:
3x − 6 = 21
Find the domain and range of the following function.
f(x) = 7x2 + 4x − 1
Check the injectivity and surjectivity of the following function.
f : R → R given by f(x) = x2
Express the following exponential equation in logarithmic form
`9^(3/2)` = 27
Express the following exponential equation in logarithmic form
e–x = 6
Solve for x.
log2 + log(x + 3) – log(3x – 5) = log3
If f(x) = 3x + 5, g(x) = 6x − 1, then find (fg) (3)
Select the correct answer from given alternatives.
Find x, if 2log2 x = 4
Select the correct answer from given alternative.
The domain and range of f(x) = 2 − |x − 5| is
Answer the following:
A function f is defined as f(x) = 4x + 5, for – 4 ≤ x < 0. Find the values of f(–1), f(–2), f(0), if they exist
Answer the following:
Solve for x, logx (8x – 3) – logx 4 = 2
Answer the following:
If `log"a"/(x + y - 2z) = log"b"/(y + z - 2x) = log"c"/(z + x - 2y)`, show that abc = 1
Answer the following:
Find (f ° g) (x) and (g ° f) (x)
f(x) = `x/(x + 1)`, g(x) = `x/(1 - x)`
A graph representing the function f(x) is given in it is clear that f(9) = 2
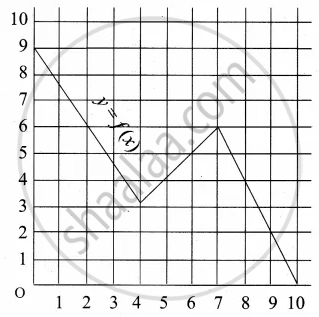
Describe the following Range
A graph representing the function f(x) is given in it is clear that f(9) = 2

What is the image of 6 under f?
A function f is defined by f(x) = 2x – 3 find `("f"(0) + "f"(1))/2`
If f(x) = 5x - 3, then f-1(x) is ______
The domain of the real valued function f(x) = `sqrt((x - 2)/(3 - x))` is ______.
Let f : R → R be defined by
f(x) = `{(3x; x > 2),(2x^2; 1 ≤ x ≤ 2), (4x; x < 1):}`
Then f(-2) + f(1) + f(3) is ______
If f(x) = `{{:(x^2",", x ≥ 0),(x^3",", x < 0):}`, then f(x) is ______.
Let f(x) = `sqrt(x)` and g(x) = x be two functions defined in the domain R+ ∪ {0}. Find `(f/g)(x)`
The domain and range of real function f defined by f(x) = `sqrt(x - 1)` is given by ______.
The domain of the function f(x) = `sin^-1((|x| + 5)/(x^2 + 1))` is (–∞, –a] ≈ [a, ∞). Then a is equal to ______.
If f(x) = `log_e{((1 - x))/((1 - x))}, |x| < 1, f{(2x)/((1 + x^2))}` is equal to ______.
The range of the function f(x) = x2 + 2x+ 2 is ______.
