Advertisements
Advertisements
प्रश्न
Find the domain of the function f(x) = `(x^2 + 2x + 1)/(x^2 - 8x + 12)`
उत्तर
The given function is `(x^2 + 2x + 1)/(x^2 - 8x + 12)`
`f(x) = (x^2 + 2x + 1)/(x^2 - 8x + 12) = (x^2 + 2x + 1)/((x - 6) (x - 2))`
It can be seen that function f is defined for all real numbers except at x = 6 and x = 2.
Hence, the domain of f(x) is R – {2, 6}.
APPEARS IN
संबंधित प्रश्न
What is the fundamental difference between a relation and a function? Is every relation a function?
Let A = {−2, −1, 0, 1, 2} and f : A → Z be a function defined by f(x) = x2 − 2x − 3. Find:
(a) range of f, i.e. f(A).
f, g, h are three function defined from R to R as follow:
(iii) h(x) = x2 + 1
Find the range of function.
Let f and g be two real functions defined by \[f\left( x \right) = \sqrt{x + 1}\] and \[g\left( x \right) = \sqrt{9 - x^2}\] . Then, describe function:
(vii) f2 + 7f
Let f and g be two real functions defined by \[f\left( x \right) = \sqrt{x + 1}\] and \[g\left( x \right) = \sqrt{9 - x^2}\] . Then, describe function:
(viii) \[\frac{5}{8}\]
If\[f\left( x \right) = 1 - \frac{1}{x}\] , then write the value of \[f\left( f\left( \frac{1}{x} \right) \right)\]
Let A and B be two sets such that n(A) = p and n(B) = q, write the number of functions from A to B.
Let f and g be two functions given by
f = {(2, 4), (5, 6), (8, −1), (10, −3)} and g = {(2, 5), (7, 1), (8, 4), (10, 13), (11, −5)}.
Find the domain of f + g
If \[e^{f\left( x \right)} = \frac{10 + x}{10 - x}\] , x ∈ (−10, 10) and \[f\left( x \right) = kf\left( \frac{200 x}{100 + x^2} \right)\] , then k =
If f(x) = sin [π2] x + sin [−π]2 x, where [x] denotes the greatest integer less than or equal to x, then
The domain of the function \[f\left( x \right) = \sqrt{\frac{\left( x + 1 \right) \left( x - 3 \right)}{x - 2}}\] is
The domain of definition of the function f(x) = log |x| is
The domain of definition of \[f\left( x \right) = \sqrt{4x - x^2}\] is
The range of the function f(x) = |x − 1| is
Check if the following relation is function:
A function f is defined as follows: f(x) = 5 − x for 0 ≤ x ≤ 4. Find the value of x such that f(x) = 3
Which sets of ordered pairs represent functions from A = {1, 2, 3, 4} to B = {−1, 0, 1, 2, 3}? Justify.
{(1, 2), (2, −1), (3, 1), (4, 3)}
If f(m) = m2 − 3m + 1, find `f(1/2)`
If f(m) = m2 − 3m + 1, find f(x + 1)
Find x, if g(x) = 0 where g(x) = 6x2 + x − 2
Express the area A of circle as a function of its diameter d
Solve for x.
log2 + log(x + 3) – log(3x – 5) = log3
If x = loga bc, y = logb ca, z = logc ab then prove that `1/(1 + x) + 1/(1 + y) + 1/(1 + z)` = 1
If f(x) = 3x + 5, g(x) = 6x − 1, then find (f + g) (x)
Select the correct answer from given alternatives.
If f : R → R is defined by f(x) = x3 then f–1 (8) is equal to :
Answer the following:
If `log (("a" + "b")/2) = 1/2(log"a" + log"b")`, then show that a = b
Answer the following:
Without using log tables, prove that `2/5 < log_10 3 < 1/2`
Answer the following:
Find value of `(3 + log_10 343)/(2 + 1/2 log_10 (49/4) + 1/2 log_10 (1/25)`
Answer the following:
Find the domain of the following function.
f(x) = 5–xPx–1
Answer the following:
Find (f ° g) (x) and (g ° f) (x)
f(x) = `x/(x + 1)`, g(x) = `x/(1 - x)`
Given the function f: x → x2 – 5x + 6, evaluate f(2a)
A graph representing the function f(x) is given in it is clear that f(9) = 2
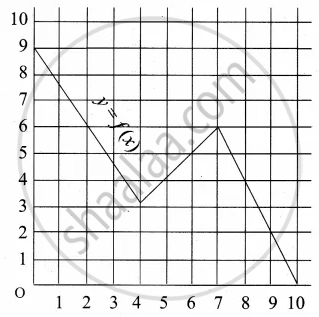
Find the following values of the function
(a) f(0)
(b) f(7)
(c) f(2)
(d) f(10)
A function f is defined by f(x) = 2x – 3 find x such that f(x) = 0
Find the domain of the following function.
f(x) = `x/(x^2 + 3x + 2)`
Find the domain of the following functions given by f(x) = x|x|
Find the range of the following functions given by f(x) = 1 + 3 cos2x
(Hint: –1 ≤ cos 2x ≤ 1 ⇒ –3 ≤ 3 cos 2x ≤ 3 ⇒ –2 ≤ 1 + 3cos 2x ≤ 4)
If f(x) = `(x - 1)/(x + 1)`, then show that `f(- 1/x) = (-1)/(f(x))`
The domain of the function f given by f(x) = `(x^2 + 2x + 1)/(x^2 - x - 6)` is ______.
The expression \[\begin{array}{cc}\log_p\log_p\sqrt[p]{\sqrt[p]{\sqrt[p]{\text{...........}\sqrt[p]{p}}}}\\
\phantom{...........}\ce{\underset{n radical signs}{\underline{\uparrow\phantom{........}\uparrow}}}
\end{array}\]where p ≥ 2, p ∈ N; ∈ N when simplified is ______.
The range of the function f(x) = `""^(7 - x)P_(x - 3)` is ______.
