Geometry Mathematics 2 Model set 1 by shaalaa.com 2021-2022 SSC (English Medium) 10th Standard Board Exam Question Paper Solution

Four alternative answers for the following question is given. Choose the correct alternative.

Two circles intersect each other such that each circle passes through the centre of the other. If the distance between their centres is 12, what is the radius of each circle?

6 cm 

12 cm 

24 cm

can’t say 

If ∆ABC ~ ∆PQR and AB : PQ = 3 : 4 then A(∆ABC) : A(∆PQR) = ?

9 : 25

9 : 16

16 : 9

25 : 9

66 cm

 44 cm

 160 cm 

 99 cm

sin²θ + sin²(90 – θ) = ?

0

1

2

`sqrt(2)`

The coordinates of diameter AB of a circle are A(2, 7) and B(4, 5), then find the coordinates of the centre

`(1 - tan^2 45^circ)/(1 + tan^2 45^circ)` = ?

Write the test of similarity for triangles given in figure.

Find distance between point A(7, 5) and B(2, 5)

The chords corresponding to congruent arcs of a circle are congruent. Prove the theorem by completing following activity.

Given: In a circle with centre B 

arc APC ≅ arc DQE

To Prove: Chord AC ≅ chord DE

Proof: In ΔABC and ΔDBE,

side AB ≅ side DB    ......`square`

side BC ≅ side `square`    .....`square`

∠ABC ≅ ∠DBE    ......[Measure of congruent arcs]

∆ABC ≅ ∆DBE    ......`square`

Complete the following activity to find the length of hypotenuse of right angled triangle, if sides of right angle are 9 cm and 12 cm.

Activity: In ∆PQR, m∠PQR = 90°

By Pythagoras Theorem,

PQ² + `square` = PR²    ......(I)

∴ PR² = 9² + 12² 

∴ PR² = `square` + 144

∴ PR² = `square`

∴ PR = 15

∴ Length of hypotenuse of triangle PQR is `square` cm.

Find distance between point Q(3, – 7) and point R(3, 3)

Solution: Suppose Q(x₁, y₁) and point R(x₂, y₂)

x₁ = 3, y₁ = – 7 and x₂ = 3, y₂ = 3

Using distance formula,

d(Q, R) = `sqrt(square)`

∴ d(Q, R) = `sqrt(square - 100)`

∴ d(Q, R) =  `sqrt(square)`

∴ d(Q, R) = `square`

ΔABP ~ ΔDEF and A(ΔABP) : A(ΔDEF) = 144:81, then AB : DE = ?

Find the volume of a cone if the radius of its base is 1.5 cm and its perpendicular height is 5 cm.

A rectangle having dimensions 35 m × 12 m, then what is the length of its diagonal?

If cos(45° + x) = sin 30°, then x = ?

An exterior angle of a cyclic quadrilateral is congruent to the angle opposite to its adjacent interior angle, to prove the theorem complete the activity.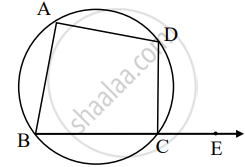

Given:  ABCD is cyclic,

`square` is the exterior angle of  ABCD

To prove: ∠DCE ≅ ∠BAD

Proof: `square` + ∠BCD = `square`    .....[Angles in linear pair] (I)

 ABCD is a cyclic.

`square` + ∠BAD = `square`     ......[Theorem of cyclic quadrilateral] (II)

By (I) and (II)

∠DCE + ∠BCD = `square` + ∠BAD

∠DCE ≅ ∠BAD

From the figure given alongside, find the length of the median AD of triangle ABC. Complete the activity.

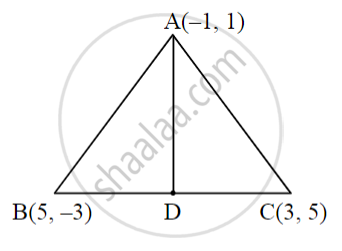
Solution:

Here A(–1, 1), B(5, – 3), C(3, 5) and suppose D(x, y) are coordinates of point D.

Using midpoint formula,

x = `(5 + 3)/2`

∴ x = `square`

y = `(-3 + 5)/2`

∴ y = `square`

Using distance formula,

∴ AD = `sqrt((4 - square)^2 + (1 - 1)^2`

∴ AD = `sqrt((square)^2 + (0)^2`

∴ AD = `sqrt(square)`

∴ The length of median AD = `square`

In ΔABC, B − D − C and BD = 7, BC = 20, then find the following ratio.

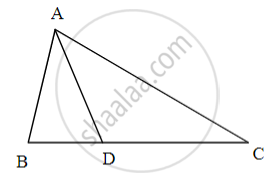
(i) `"A(ΔABD)"/"A(ΔADC)"`

(ii) `"A(ΔABD)"/"A(ΔABC)"`

(iii) `"A(ΔADC)"/"A(ΔABC)"`

The radii of ends of a frustum are 14 cm and 6 cm respectively and its height is 6 cm. Find its volume \[\pi\] = 3.14) 

A congruent side of an isosceles right angled triangle is 7 cm, Find its perimeter

Construct an equilateral ∆ABC with side 5 cm. ∆ABC ~ ∆LMN, ratio the corresponding sides of triangle is 6 : 7, then construct ΔLMN and ΔABC

In the figure, ▢ABCD is a cyclic quadrilateral. If m(arc ABC) = 230°, then find ∠ABC, ∠CDA, ∠CBE.

A solid consisting of a right circular cone of height 120 cm and radius 60 cm standing on a hemisphere of radius 60 cm is placed upright in a right circular cylinder full of water such that it touches the bottom. Find the volume of water left in the cylinder, if the radius of the cylinder is 60 cm and its height is 180 cm. Use [π = `22/7`]

ΔAMT ~ ΔAHE. In ΔAMT, AM = 6.3 cm, ∠MAT = 120°, AT = 4.9 cm, `"AM"/"HA" = 7/5`, then construct ΔAMT and ΔAHE

In fig., PS = 2, SQ = 6, QR = 5, PT = x and TR = y. Then find the pair of value of x and y such that ST || side QR.

In ∆ABC, cos C = `12/13` and BC = 24, then AC = ?