Advertisements
Advertisements
प्रश्न
ABC is a right triangle with AB = AC. Bisector of ∠A meets BC at D. Prove that BC = 2AD.
उत्तर
We have given, ΔABC which is an isosceles right triangle with AB = AC and AD is the bisector of ∠A.
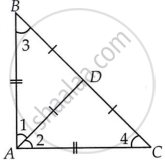
Now in ΔABC,
AB = AC ...[Given]
⇒ ∠C = ∠B ...(1) [Angles opposite to equal sides are equal]
Now, in ΔABC, ∠A = 90°
∠A + ∠B + ∠C = 180° ...[Angle sum property of Δ]
⇒ 90° + ∠B + ∠B = 180° ...[From (1)]
⇒ 2∠B = 90°
⇒ ∠B = 45°
⇒ ∠B = ∠C = 45° or ∠3 = ∠4 = 45°
Also, ∠1 = ∠2 = 45° ...[∵ AD is bisector of ∠A]
Also, ∠1 = ∠3, ∠2 = ∠4 = 45°
⇒ BD = AD, DC = AD ...(2) [Sides opposite to equal angles are equal]
Thus, BC = BD + DC = AD + AD ...[From (2)]
⇒ BC = 2AD
APPEARS IN
संबंधित प्रश्न
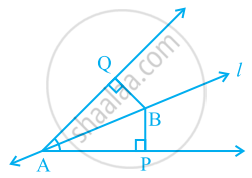
Line l is the bisector of an angle ∠A and B is any point on l. BP and BQ are perpendiculars from B to the arms of ∠A (see the given figure). Show that:
- ΔAPB ≅ ΔAQB
- BP = BQ or B is equidistant from the arms of ∠A.
Which congruence criterion do you use in the following?
Given: AC = DF
AB = DE
BC = EF
So, ΔABC ≅ ΔDEF

You want to show that ΔART ≅ ΔPEN,
If it is given that ∠T = ∠N and you are to use SAS criterion, you need to have
1) RT = and
2) PN =

In two congruent triangles ABC and DEF, if AB = DE and BC = EF. Name the pairs of equal angles.
In two triangles ABC and DEF, it is given that ∠A = ∠D, ∠B = ∠E and ∠C =∠F. Are the two triangles necessarily congruent?
If ABC and DEF are two triangles such that AC = 2.5 cm, BC = 5 cm, ∠C = 75°, DE = 2.5 cm, DF = 5cm and ∠D = 75°. Are two triangles congruent?
From the given diagram, in which ABCD is a parallelogram, ABL is a line segment and E is mid-point of BC.
Prove that:
(i) ΔDCE ≅ ΔLBE
(ii) AB = BL.
(iii) AL = 2DC
In ∆ABC, AB = AC. Show that the altitude AD is median also.
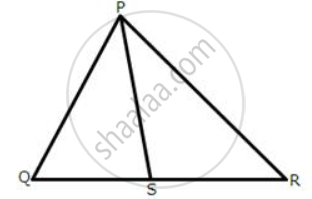
In the following figures, the sides AB and BC and the median AD of triangle ABC are equal to the sides PQ and QR and median PS of the triangle PQR.
Prove that ΔABC and ΔPQR are congruent.
 |
 |
In the following figure, ∠A = ∠C and AB = BC.
Prove that ΔABD ≅ ΔCBE. 
