Advertisements
Advertisements
प्रश्न
Find x and y, in each of the following figure: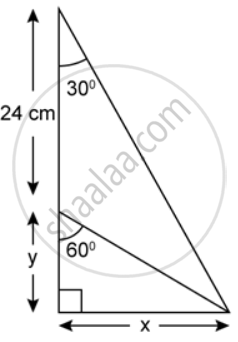
उत्तर

In right ΔABC,
tan30° = `"BC"/"AB"`
⇒ `(1)/sqrt(3) = x/(24 + y)` ....(i)
In right ΔDBC,
tan60° = `"BC"/"DB"`
⇒ `sqrt(3) = x/y`
⇒ x = `sqrt(3)y`
Substituting the value of x in (i), we get
`(1)/sqrt(3) = sqrt(3)/(24 + y)`
⇒ 24 + y = 3y
⇒ 2y = 24
⇒ y = 12cm
⇒ x = `sqrt(3) xx 12 = 12sqrt(3)"cm"`.
APPEARS IN
संबंधित प्रश्न
From the given figure,
find:
(i) cos x°
(ii) x°
(iii) `(1)/(tan^2 xx°) – (1)/(sin^2xx°)`
(iv) Use tan xo, to find the value of y.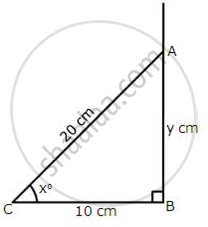
Solve for x : sin (x + 10°) = `(1)/(2)`
Solve for x : cos2 30° + sin2 2x = 1
Solve for x : sin2 60° + cos2 (3x- 9°) = 1
Solve for 'θ': cot2(θ - 5)° = 3
If A = B = 60°, verify that: tan(A - B) = `(tan"A" - tan"B")/(1 + tan"A" tan"B"")`
In a right triangle ABC, right angled at C, if ∠B = 60° and AB = 15units, find the remaining angles and sides.
Find lengths of diagonals AC and BD. Given AB = 24 cm and ∠BAD = 60°.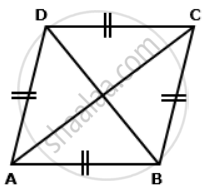
Evaluate the following: `(sin25° cos43°)/(sin47° cos 65°)`
Evaluate the following: tan(78° + θ) + cosec(42° + θ) - cot(12° - θ) - sec(48° - θ)
