Advertisements
Advertisements
प्रश्न
How many time constants will elapse before the current in a charging RC circuit drops to half of its initial value? Answer the same question for a discharging RC circuit.
उत्तर
The growth of charge across a capacitor,
\[q = Q\left( 1 - e^{- \frac{t}{RC}} \right)\]
\[q = \frac{Q}{2}\]
\[ \Rightarrow \frac{Q}{2} = Q \left( 1 - e^{- \frac{t}{RC}} \right)\]
\[ \Rightarrow \frac{1}{2} = \left( 1 - e^{- \frac{t}{RC}} \right)\]
\[ \Rightarrow e^{- \frac{t}{RC}} = \frac{1}{2}\]
\[ \Rightarrow \frac{t}{RC} = \ln 2 \]
Let t = nRC
\[ \Rightarrow \frac{nRC}{RC} = 0 . 69\]
\[ \Rightarrow n = 0 . 69\]
The decay of charge across a capacitor,
\[q = Q e^{- \frac{t}{RC}} \]
\[q = \frac{Q}{2}\]
\[ \Rightarrow \frac{Q}{2} = Q e^{- \frac{t}{RC}} \]
\[ \Rightarrow \frac{1}{2} = e^{- \frac{t}{RC}} \]
Let t = nRC
\[ \Rightarrow \frac{nRC}{RC} = \ln 2\]
\[ \Rightarrow n = 0 . 69\]
APPEARS IN
संबंधित प्रश्न
Obtain the expression for the energy stored per unit volume in a charged parallel plate capacitor.
A 12 pF capacitor is connected to a 50 V battery. How much electrostatic energy is stored in the capacitor?
A 600 pF capacitor is charged by a 200 V supply. It is then disconnected from the supply and is connected to another uncharged 600 pF capacitor. How much electrostatic energy is lost in the process?
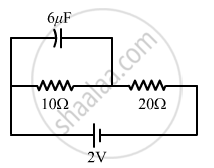
Find the charge on the capacitor as shown in the circuit.
The energy density in the electric field created by a point charge falls off with the distance from the point charge as
Find the charge on the capacitor shown in the figure.

A capacitance C, a resistance R and an emf ε are connected in series at t = 0. What is the maximum value of (a) the potential difference across the resistor (b) the current in the circuit (c) the potential difference across the capacitor (d) the energy stored in the capacitor (e) the power delivered by the battery and (f) the power converted into heat?
Two capacitors of capacitances 4⋅0 µF and 6⋅0 µF are connected in series with a battery of 20 V. Find the energy supplied by the battery.
A capacitor of capacitance C is connected to a battery of emf ε at t = 0 through a resistance R. Find the maximum rate at which energy is stored in the capacitor. When does the rate have this maximum value?
A capacitor of capacitance 12.0 μF is connected to a battery of emf 6.00 V and internal resistance 1.00 Ω through resistanceless leads. 12.0 μs after the connections are made, what will be (a) the current in the circuit (b) the power delivered by the battery (c) the power dissipated in heat and (d) the rate at which the energy stored in the capacitor is increasing?
A capacitance C charged to a potential difference V is discharged by connecting its plates through a resistance R. Find the heat dissipated in one time constant after the connections are made. Do this by calculating ∫ i2R dt and also by finding the decrease in the energy stored in the capacitor.
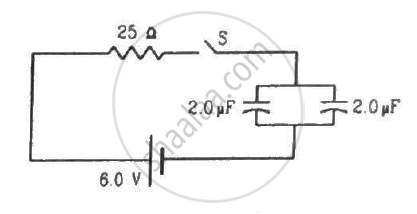
Find the charge on each of the capacitors 0.20 ms after the switch S is closed in the figure.
Each capacitor in figure has a capacitance of 10 µF. The emf of the battery is 100 V. Find the energy stored in each of the four capacitors.
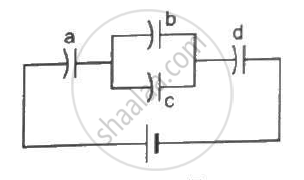
A capacitor with stored energy 4⋅0 J is connected with an identical capacitor with no electric field in between. Find the total energy stored in the two capacitors.
A capacitor of capacitance C is given a charge Q. At t = 0, it is connected to an ideal battery of emf ε through a resistance R. Find the charge on the capacitor at time t.
A capacitor is a device that stores ____________.
Prove that, if an insulated, uncharged conductor is placed near a charged conductor and no other conductors are present, the uncharged body must be intermediate in potential between that of the charged body and that of infinity.
A fully charged capacitor C with initial charge q0 is connected to a coil of self-inductance L at t = 0. The time at which the energy is stored equally between the electric and magnetic fields is ______.
In a capacitor of capacitance 20 µF, the distance between the plates is 2 mm. If a dielectric slab of width 1 mm and dielectric constant 2 is inserted between the plates, what is the new capacitance?
