Advertisements
Advertisements
प्रश्न
If θ = 30°, verify that: sin 3θ = 4sinθ . sin(60° - θ) sin(60° + θ)
उत्तर
Given: θ = 30°
sin3θ
= sin3 x 30°
= sin90°
= 1
4sinθ . sin(60° - θ) sin(60° + θ)
= 4sin 30° x sin(60° - 30°) x sin(60° + 30°)
= 4sin30° x sin30° x sin90°
= `4 xx (1)/(2) xx (1)/(2) xx 1`
= 1
⇒ sin3θ = 4sinθ . sin(60° - θ)sin(60° + θ).
APPEARS IN
संबंधित प्रश्न
If 4 cos2 x° - 1 = 0 and 0 ∠ x° ∠ 90°,
find:(i) x°
(ii) sin2 x° + cos2 x°
(iii) `(1)/(cos^2xx°) – (tan^2 xx°)`
Find the magnitude of angle A, if tan A - 2 cos A tan A + 2 cos A - 1 = 0
In the given figure, PQ = 6 cm, RQ = x cm and RP = 10 cm, find
a. cosθ
b. sin2θ- cos2θ
c. Use tanθ to find the value of RQ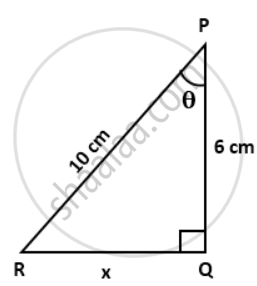
In a right triangle ABC, right angled at C, if ∠B = 60° and AB = 15units, find the remaining angles and sides.
In a trapezium ABCD, as shown, AB ‖ DC, AD = DC = BC = 24 cm and ∠A = 30°. Find: length of AB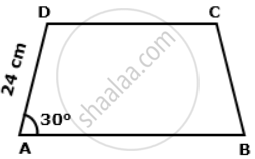
In the given figure, AB and EC are parallel to each other. Sides AD and BC are 1.5 cm each and are perpendicular to AB. Given that ∠AED = 45° and ∠ACD = 30°. Find:
a. AB
b. AC
c. AE
Find the value 'x', if: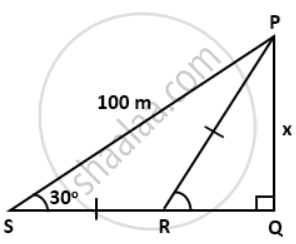
Express each of the following in terms of trigonometric ratios of angles between 0° and 45°: sin53° + sec66° - sin50°
Evaluate the following: `(2sin25° sin35° sec55° sec65°)/(5tan 29° tan45° tan61°) + (3cos20° cos50° cot70° cot40°)/(5tan20° tan50° sin70° sin40°)`
If sec2θ = cosec3θ, find the value of θ if it is known that both 2θ and 3θ are acute angles.
