Advertisements
Advertisements
प्रश्न
If sin θ ,` sqrt (3)/2` find the value of all T- ratios of θ .
उत्तर
Let us first draw a right ΔABC, right angled at B and ∠𝐶 = 𝜃
Now, we know that sin 𝜃 = `"𝑃𝑟𝑒𝑝𝑒𝑛𝑑𝑖𝑐𝑢𝑙𝑎𝑟 "/"ℎ𝑦𝑝𝑜𝑡𝑒𝑛𝑢𝑠𝑒" = (AB)/ (AC) = sqrt(3)/2`
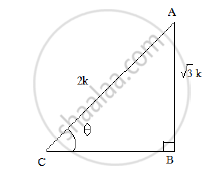
So, if AB = `sqrt(3)`𝑘, 𝑡ℎ𝑒𝑛 𝐴𝐶 = 2𝑘, 𝑤ℎ𝑒𝑟𝑒 𝑘 𝑖𝑠 𝑎 𝑝𝑜𝑠𝑖𝑡𝑖𝑣𝑒 𝑛𝑢𝑚𝑏𝑒𝑟.
Now, using Pythagoras theorem, we have:
`AC^2 = AB^2 + BC^2`
`⟹ BC^2 = AC^2 − AB^2 = (2K)^2 − (sqrt(3)K)^2`
`⟹ BC^2 = 4K^2 − 3K^2 = K^2`
⟹ BC = k
Now, finding the other T-rations using their definitions, we get:
Cos 𝜃 =` (BC)/(AC) = K/(2K)= 1/2`
Tan 𝜃 =`(AB)/(BC) = (sqrt(3K))/K = sqrt(3)`
∴ cot 𝜃 =`1/(Tan θ ) = 1/sqrt(3), cosec θ = 1/(sin θ) = 2/(sqrt(3)) and sec θ = 1/ (cos θ) = 2 `
APPEARS IN
संबंधित प्रश्न
if `sin theta = 3/5 " evaluate " (cos theta - 1/(tan theta))/(2 cot theta)`
In right angled triangle ΔABC at B, ∠A = ∠C. Find the values of sin A sin B + cos A cos B
If ∠A and ∠B are acute angles such that sin A = Sin B prove that ∠A = ∠B.
If A = 300 , verify that:
(iii) tan 2A = `(2tanA)/(1-tan^2A)`
Using the formula, tan 2A =`(2 tan A )/(1- tan^2 A)` find the value of tan 600, it being given that tan 300 = `1/sqrt(3)`.
Using the formula, cos A = `sqrt((1+cos2A)/2) ,`find the value of cos 300, it being given that cos 600 = `1/2`.
Given: sec A = `( 29 )/(21), "evaluate : sin A" - 1/tan "A"`
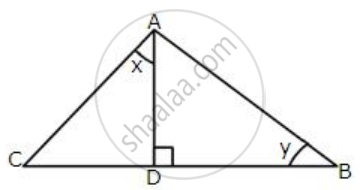
In the following figure:
AD ⊥ BC, AC = 26 CD = 10, BC = 42, ∠DAC = x and ∠B = y.
Find the value of :
(i) cot x
(ii) `1/sin^2 y – 1/tan^2 y`
(iii) `6/cos x – 5/cos y + 8 tan y`.
In rhombus ABCD, diagonals AC and BD intersect each other at point O.
If cosine of angle CAB is 0.6 and OB = 8 cm, find the lengths of the side and the diagonals of the rhombus.
In the given figure, ΔABC is right angled at B.AD divides BC in the ratio 1 : 2. Find
(i) `("tan"∠"BAC")/("tan"∠"BAD")` (ii) `("cot"∠"BAC")/("cot"∠"BAD")`
