Advertisements
Advertisements
प्रश्न
In a cyclic quadrilateral ABCD, ∠A : ∠C = 3 : 1 and ∠B : ∠D = 1 : 5; find each angle of the quadrilateral.
उत्तर
Let ∠A and ∠C be 3x and x respectively
In cyclic quadrilateral ABCD,
∠A + ∠C = 180°
(Pairs of opposite angles in a cyclic quadrilateral are supplementary)
`=>` 3x + x = 180°
`=> x = (180^circ)/4 = 45^circ`
∴ ∠A = 135° and ∠C = 45°
Let the measure of ∠B and ∠D be y and 5y respectively
In cyclic quadrilateral ABCD,
∠B + ∠D = 180°
(Pair of opposite angles in a cyclic quadrilateral are supplementary are supplementary)
`=>` y + 5y = 180°
`=> y = (180^circ)/6 = 30^circ`
∴ ∠B = 30° and ∠D = 150°
APPEARS IN
संबंधित प्रश्न
ABCD is a quadrilateral inscribed in a circle, having ∠ = 60°; O is the center of the circle.
Show that: ∠OBD + ∠ODB =∠CBD +∠CDB.
ABCD is a cyclic quadrilateral in which AB and DC on being produced, meet at P such that PA = PD. Prove that AD is parallel to BC.
In the given figure, SP is bisector of ∠RPT and PQRS is a cyclic quadrilateral. Prove that : SQ = SR.

ABCDE is a cyclic pentagon with centre of its circumcircle at point O such that AB = BC = CD and angle ABC = 120°.
Calculate:
- ∠BEC
- ∠BED
D and E are points on equal sides AB and AC of an isosceles triangle ABC such that AD = AE. Prove that the points B, C, E and D are concyclic.
Two circles intersect in points P and Q. A secant passing through P intersects the circles in A and B respectively. Tangents to the circles at A and B intersect at T. Prove that A, Q, B and T lie on a circle.
In following figure , O is the centre of the circle. If ∠ APB = 50° then find ∠ AOB and ∠ OAB.

In triangle ABC, AB = AC. A circle passing through B and c intersects the sides AB and AC at D and E respectively. Prove that DE || BC.
Prove that the angle bisectors of the angles formed by producing opposite sides of a cyclic quadrilateral (Provided they are not parallel) intersect at the right angle.
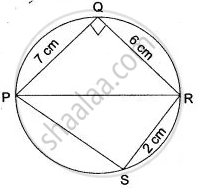
In the figure alongside PR is a diameter of the circle, PQ = 7 cm; QR = 6 cm and RS = 2 cm. Calculate the perimeter of the cyclic quadrilateral PQRS.