Advertisements
Advertisements
प्रश्न
In a triangle ABC right angled at C, P and Q are points of sides CA and CB respectively, which divide these sides the ratio 2 : 1.
Prove that : 9(AQ2 + BP2) = 13AB2
उत्तर
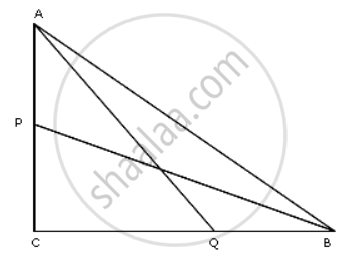
P divides AC in the ratio 2 : 1
So C.P. = `(2)/(3) "AC"` .......(i)
Q divides BC in the ratio 2 : 1
QC = `(2)/(3)"BC"` ......(ii)
Adding (iii) and (iv), we get
9(AQ2 + BP2) = 13(BC2 + AC2)
⇒ 9(AQ2 + BP2) = 13AB2.
APPEARS IN
संबंधित प्रश्न
Side of a triangle is given, determine it is a right triangle.
`(2a – 1) cm, 2\sqrt { 2a } cm, and (2a + 1) cm`
In figure, ∠B of ∆ABC is an acute angle and AD ⊥ BC, prove that AC2 = AB2 + BC2 – 2BC × BD
P and Q are the mid-points of the sides CA and CB respectively of a ∆ABC, right angled at C. Prove that:
`(i) 4AQ^2 = 4AC^2 + BC^2`
`(ii) 4BP^2 = 4BC^2 + AC^2`
`(iii) (4AQ^2 + BP^2 ) = 5AB^2`
ABC is an isosceles triangle right angled at C. Prove that AB2 = 2AC2
A guy wire attached to a vertical pole of height 18 m is 24 m long and has a stake attached to the other end. How far from the base of the pole should the stake be driven so that the wire will be taut?
PQR is a triangle right angled at P. If PQ = 10 cm and PR = 24 cm, find QR.
Identify, with reason, if the following is a Pythagorean triplet.
(4, 9, 12)
In ∆PQR, point S is the midpoint of side QR. If PQ = 11, PR = 17, PS = 13, find QR.
Digonals of parallelogram WXYZ intersect at point O. If OY =5, find WY.
A man goes 40 m due north and then 50 m due west. Find his distance from the starting point.
In triangle ABC, given below, AB = 8 cm, BC = 6 cm and AC = 3 cm. Calculate the length of OC.

Find the length of diagonal of the square whose side is 8 cm.
Find the value of (sin2 33 + sin2 57°)
Find the Pythagorean triplet from among the following set of numbers.
4, 5, 6
In a triangle ABC, AC > AB, D is the midpoint BC, and AE ⊥ BC. Prove that: AB2 + AC2 = 2(AD2 + CD2)
In a triangle ABC right angled at C, P and Q are points of sides CA and CB respectively, which divide these sides the ratio 2 : 1.
Prove that: 9AQ2 = 9AC2 + 4BC2
In a right angled triangle, the hypotenuse is the greatest side
In the adjoining figure, a tangent is drawn to a circle of radius 4 cm and centre C, at the point S. Find the length of the tangent ST, if CT = 10 cm.

Lengths of sides of a triangle are 3 cm, 4 cm and 5 cm. The triangle is ______.
Two rectangles are congruent, if they have same ______ and ______.
