Advertisements
Advertisements
प्रश्न
In each of the following, one trigonometric ratio is given. Find the values of the other trigonometric.
cos A = `(7)/(25)`
उत्तर
cos A = `(7)/(25)`
cosA = `"Base"/"Hypotenuse" = (7)/(25)`
By Pythagoras theorem, we have
(Hypotenuse)2 = (Perpendicular)2 + (Base)2
⇒ Perpendicular = `sqrt(("Hypotenuse")^2 - ("Base")^2`
⇒ Perpendicular
= `sqrt((25)^2 - (7)^2`
= `sqrt(625 - 49)`
= `sqrt(576)`
= 24
sinA = `"Perpendicular"/"Hypotenuse" = (24)/(25)`
tanA = `"Perpendicular"/"Base" = (24)/(7)`
secA = `(1)/"cosA" = (25)/(7)`
cotA = `(1)/"tanA" = (7)/(24)`
cosecA = `(1)/"sinA" = (25)/(24)`.
APPEARS IN
संबंधित प्रश्न
In ∆PQR, right-angled at Q, PQ = 3 cm and PR = 6 cm. Determine ∠P and ∠R.
If sin A = `9/41` find all the values of cos A and tan A
If sec `theta = 17/8 ` verify that `((3-4sin^2theta)/(4 cos^2theta -3))=((3-tan^2theta)/(1-tan^2theta))`
If A = 600 and B = 300, verify that:
(i) sin (A – B) = sin A cos B – cos A sin B
Using the formula, cos A = `sqrt((1+cos2A)/2) ,`find the value of cos 300, it being given that cos 600 = `1/2`.
Given: sec A = `( 29 )/(21), "evaluate : sin A" - 1/tan "A"`
Given: sin θ = `p/q`.
Find cos θ + sin θ in terms of p and q.
If cosec θ = `sqrt5`, find the value of:
- 2 - sin2 θ - cos2 θ
- 2 + `1/sin^2"θ" – cos^2"θ"/sin^2"θ"`
If sec A = `sqrt2` , find : `(3cot^2 "A"+ 2 sin^2 "A")/ (tan^2 "A" – cos ^2 "A")`.
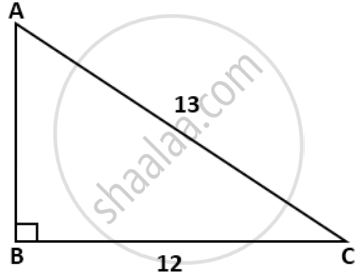
In the given figure, AC = 13cm, BC = 12 cm and ∠B = 90°. Without using tables, find the values of: `("cos A" - "sin A")/("cos A" + "sin A")`