Advertisements
Advertisements
प्रश्न
In the given figure, ABCD is a cyclic quadrilateral. If ∠BCD = 100° and ∠ABD = 70°, find ∠ADB.
Advertisements
उत्तर
It is given that ∠BCD = 100° and ∠ABD = 70°
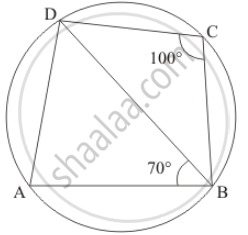
We have to find the ∠ADB
We have
∠A + ∠C = 180° (Opposite pair of angle of cyclic quadrilateral)
So,
`angle A = 180° - 100°`
= 80°
Now in Δ ADB is `angle A ` = 80° and `angle ABD` = 70°
Therefore,
`angle A + angle ADB + angle ABD = 180°`
`80° + angleADB + 70° = 180°`
`angleADB = 180° - 150°`
= 30°
Hence, `angleADB` = 30°
APPEARS IN
संबंधित प्रश्न
In Fig. 1, QR is a common tangent to the given circles, touching externally at the point T. The tangent at T meets QR at P. If PT = 3.8 cm, then the length of QR (in cm) is :
(A) 3.8
(B) 7.6
(C) 5.7
(D) 1.9
Suppose You Are Given a Circle. Give a Construction to Find Its Centre.
From an external point P, tangents PA and PB are drawn to a circle with center O. If CD is the tangent to the circle at a point E and PA = 14cm, find the perimeter of ΔPCD.
In the given figure, O is the centre of the circle. PA and PB are tangents. Show that AOBP is cyclic quadrilateral.
Tangents PA and PB are drawn from an external point P to two concentric circles with centre O and radii 8 cm and 5 cm respectively, as shown in Fig. 3. If AP = 15 cm, then find the length of BP.
In the given figure, ABC is a right triangle right-angled at B such that BC = 6 cm and AB = 8 cm. Find the radius of its incircle.
Find the area of the shaded region in the figure If ABCD is a rectangle with sides 8 cm and 6 cm and O is the centre of the circle. (Take π= 3.14)
A point A is 26 cm away from the centre of a circle and the length of the tangent drawn from A to the circle is 24 cm. Find the radius of the circle.
The tangent at a point C of a circle and a diameter AB when extended intersect at P. If ∠PCA = 110°, find ∠CBA see figure
AD is a diameter of a circle and AB is a chord. If AD = 34 cm, AB = 30 cm, the distance of AB from the centre of the circle is ______.
