Question

Consider the situation of the previous problem. The man has to reach the other shore at the point directly opposite to his starting point. If he reaches the other shore somewhere else, he has to walk down to this point. Find the minimum distance that he has to walk. 

Answer 1

Given:
Width of the river = 500 m
Rate of flow of the river = 5 km/h
Swimmer's speed with respect to water = 3 km/h
As per the question, the man has to reach the other shore at the point directly opposite to his starting point.

 

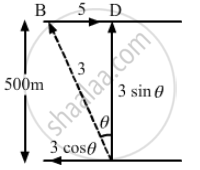
Horizontal distance is BD for the resultant velocity v_r.
x-component of the resultant velocity, R = 5 - 3 cos θ
Vertical component of velocity = 3 sin θ km/h

\[\text{ Time }  = \frac{\text{ Distance } }{\text{ Velocity } } = \frac{0 . 5}{3\sin\theta} h\]

This is the same as the horizontal component of velocity.
Thus, we have:

\[BD = \left( 5 - 3 \cos \theta \right) \left( \frac{0 . 5}{3\sin \theta} \right)\]

\[ = \frac{5 - 3 \cos \theta}{6 \sin \theta}\]

For H (horizontal distance) to be minimum,

\[\left( \frac{dH}{d\theta} \right) = 0\]

\[\Rightarrow \frac{d}{d\theta}\left( \frac{5 + 3 \cos \theta}{6 \sin \theta} \right) = 0\]

⇒ 18 (sin² θ + cos² θ) + 30 cos θ = 0
⇒ −30 cos θ = 18

\[\Rightarrow \cos \theta = - \frac{18}{30} = - \frac{3}{5}\]

\[\text{ Negative sign shows that }  \theta \text{ lies in the 2nd Quadrant .}  \]

\[ \text{ And }, \]

\[\sin \theta = \sqrt{1 - \cos^2 \theta } = \frac{4}{5}\]

\[ \therefore H = \frac{5 - 3 \cos \theta}{6 \sin \theta}\]

\[ = \frac{5 - 3\left( \frac{3}{5} \right)}{6 \times \frac{4}{5}} = \frac{25 - 9}{24}\]

\[ = \frac{16}{24} = \frac{2}{3} \text{ km }\]