Advertisements
Advertisements
Question
Find the equation of a straight line with slope − 1/3 and y-intercept − 4.
Solution
Here, \[m = - \frac{1}{3}, c = - 4\]
Substituting the values of m and c in y = mx + c, we get,
\[y = - \frac{x}{3} - 4\]
\[ \Rightarrow x + 3y + 12 = 0\]
Hence, the equation of the straight line with slope
APPEARS IN
RELATED QUESTIONS
Find a point on the x-axis, which is equidistant from the points (7, 6) and (3, 4).
Find the slope of a line, which passes through the origin, and the mid-point of the line segment joining the points P (0, –4) and B (8, 0).
Find the value of x for which the points (x, –1), (2, 1) and (4, 5) are collinear.
A line passes through (x1, y1) and (h, k). If slope of the line is m, show that k – y1 = m (h – x1).
Find the slope of a line passing through the following point:
(3, −5), and (1, 2)
State whether the two lines in each of the following are parallel, perpendicular or neither.
Through (9, 5) and (−1, 1); through (3, −5) and (8, −3)
Using the method of slope, show that the following points are collinear A (4, 8), B (5, 12), C (9, 28).
What is the value of y so that the line through (3, y) and (2, 7) is parallel to the line through (−1, 4) and (0, 6)?
What can be said regarding a line if its slope is positive ?
The slope of a line is double of the slope of another line. If tangents of the angle between them is \[\frac{1}{3}\],find the slopes of the other line.
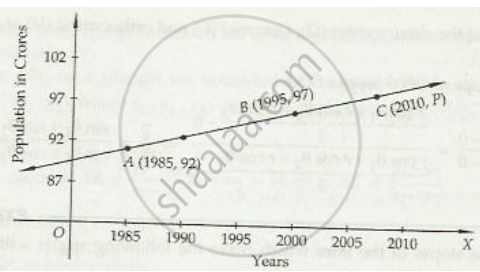
Consider the following population and year graph:
Find the slope of the line AB and using it, find what will be the population in the year 2010.
Find the angle between the X-axis and the line joining the points (3, −1) and (4, −2).
Find the equations of the bisectors of the angles between the coordinate axes.
Find the equation of the perpendicular to the line segment joining (4, 3) and (−1, 1) if it cuts off an intercept −3 from y-axis.
If the image of the point (2, 1) with respect to a line mirror is (5, 2), find the equation of the mirror.
Find the equation of the right bisector of the line segment joining the points (3, 4) and (−1, 2).
Find the angles between the following pair of straight lines:
3x − y + 5 = 0 and x − 3y + 1 = 0
Find the angles between the following pair of straight lines:
x − 4y = 3 and 6x − y = 11
Find the angles between the following pair of straight lines:
(m2 − mn) y = (mn + n2) x + n3 and (mn + m2) y = (mn − n2) x + m3.
Prove that the points (2, −1), (0, 2), (2, 3) and (4, 0) are the coordinates of the vertices of a parallelogram and find the angle between its diagonals.
Find the angle between the line joining the points (2, 0), (0, 3) and the line x + y = 1.
If θ is the angle which the straight line joining the points (x1, y1) and (x2, y2) subtends at the origin, prove that \[\tan \theta = \frac{x_2 y_1 - x_1 y_2}{x_1 x_2 + y_1 y_2}\text { and } \cos \theta = \frac{x_1 x_2 + y_1 y_2}{\sqrt{{x_1}^2 + {y_1}^2}\sqrt{{x_2}^2 + {y_2}^2}}\].
The acute angle between the medians drawn from the acute angles of a right angled isosceles triangle is
The reflection of the point (4, −13) about the line 5x + y + 6 = 0 is
Find the equation to the straight line passing through the point of intersection of the lines 5x – 6y – 1 = 0 and 3x + 2y + 5 = 0 and perpendicular to the line 3x – 5y + 11 = 0.
If one diagonal of a square is along the line 8x – 15y = 0 and one of its vertex is at (1, 2), then find the equation of sides of the square passing through this vertex.
The coordinates of the foot of the perpendicular from the point (2, 3) on the line x + y – 11 = 0 are ______.
Equation of the line passing through (1, 2) and parallel to the line y = 3x – 1 is ______.
The point (4, 1) undergoes the following two successive transformations:
(i) Reflection about the line y = x
(ii) Translation through a distance 2 units along the positive x-axis Then the final coordinates of the point are ______.
Equations of the lines through the point (3, 2) and making an angle of 45° with the line x – 2y = 3 are ______.
The points (3, 4) and (2, – 6) are situated on the ______ of the line 3x – 4y – 8 = 0.
The points A(– 2, 1), B(0, 5), C(– 1, 2) are collinear.
The vertex of an equilateral triangle is (2, 3) and the equation of the opposite side is x + y = 2. Then the other two sides are y – 3 = `(2 +- sqrt(3)) (x - 2)`.
| Column C1 | Column C2 |
| (a) The coordinates of the points P and Q on the line x + 5y = 13 which are at a distance of 2 units from the line 12x – 5y + 26 = 0 are |
(i) (3, 1), (–7, 11) |
| (b) The coordinates of the point on the line x + y = 4, which are at a unit distance from the line 4x + 3y – 10 = 0 are |
(ii) `(- 1/3, 11/3), (4/3, 7/3)` |
| (c) The coordinates of the point on the line joining A (–2, 5) and B (3, 1) such that AP = PQ = QB are |
(iii) `(1, 12/5), (-3, 16/5)` |
The equation of the line through the intersection of the lines 2x – 3y = 0 and 4x – 5y = 2 and
| Column C1 | Column C2 |
| (a) Through the point (2, 1) is | (i) 2x – y = 4 |
| (b) Perpendicular to the line (ii) x + y – 5 = 0 x + 2y + 1 = 0 is |
(ii) x + y – 5 = 0 |
| (c) Parallel to the line (iii) x – y –1 = 0 3x – 4y + 5 = 0 is |
(iii) x – y –1 = 0 |
| (d) Equally inclined to the axes is | (iv) 3x – 4y – 1 = 0 |
The line which passes through the origin and intersect the two lines `(x - 1)/2 = (y + 3)/4 = (z - 5)/3, (x - 4)/2 = (y + 3)/3 = (z - 14)/4`, is ______.
