Advertisements
Advertisements
Question
Find the image of the point (3, 8) with respect to the line x + 3y = 7 assuming the line to be a plane mirror.
Solution
Let the image of A (3,8) be B (a,b). Also, let M be the midpoint of AB.
\[\therefore\text { Coordinates of M } = \left( \frac{3 + a}{2}, \frac{8 + b}{2} \right)\]
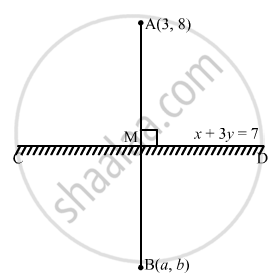
Point M lies on the line x + 3y = 7
\[\therefore \frac{3 + a}{2} + 3 \times \left( \frac{8 + b}{2} \right) = 7\]
\[\Rightarrow a + 3b + 13 = 0\] ... (1)
Lines CD and AB are perpendicular.
∴ Slope of AB \[\times\] Slope of CD = −1
\[\Rightarrow \frac{b - 8}{a - 3} \times \left( - \frac{1}{3} \right) = - 1\]
\[ \Rightarrow b - 8 = 3a - 9\]
\[\Rightarrow 3a - b - 1 = 0\] ... (2)
Solving (1) and (2) by cross multiplication, we get:
\[\frac{a}{- 3 + 13} = \frac{b}{39 + 1} = \frac{1}{- 1 - 9}\]
\[ \Rightarrow a = - 1, b = - 4\]
Hence, the image of the point (3, 8) with respect to the line mirror x + 3y = 7 is (−1, −4).
APPEARS IN
RELATED QUESTIONS
Find the slope of the line, which makes an angle of 30° with the positive direction of y-axis measured anticlockwise.
Find the equation of a line drawn perpendicular to the line `x/4 + y/6 = 1`through the point, where it meets the y-axis.
Find the slope of the lines which make the following angle with the positive direction of x-axis: \[\frac{\pi}{3}\]
What can be said regarding a line if its slope is zero ?
Show that the line joining (2, −3) and (−5, 1) is parallel to the line joining (7, −1) and (0, 3).
Show that the line joining (2, −5) and (−2, 5) is perpendicular to the line joining (6, 3) and (1, 1).
The slope of a line is double of the slope of another line. If tangents of the angle between them is \[\frac{1}{3}\],find the slopes of the other line.
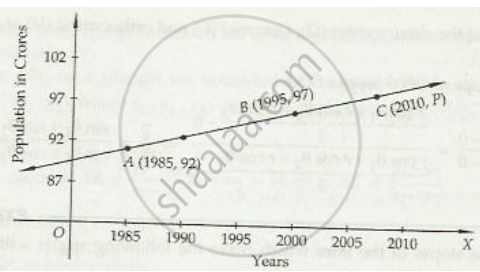
Consider the following population and year graph:
Find the slope of the line AB and using it, find what will be the population in the year 2010.
Line through the points (−2, 6) and (4, 8) is perpendicular to the line through the points (8, 12) and (x, 24). Find the value of x.
Find the angle between X-axis and the line joining the points (3, −1) and (4, −2).
Find the equation of the strainght line intersecting y-axis at a distance of 2 units above the origin and making an angle of 30° with the positive direction of the x-axis.
Find the equations of the straight lines which cut off an intercept 5 from the y-axis and are equally inclined to the axes.
Find the angles between the following pair of straight lines:
3x + 4y − 7 = 0 and 4x − 3y + 5 = 0
Find the acute angle between the lines 2x − y + 3 = 0 and x + y + 2 = 0.
Prove that the points (2, −1), (0, 2), (2, 3) and (4, 0) are the coordinates of the vertices of a parallelogram and find the angle between its diagonals.
Prove that the straight lines (a + b) x + (a − b ) y = 2ab, (a − b) x + (a + b) y = 2ab and x + y = 0 form an isosceles triangle whose vertical angle is 2 tan−1 \[\left( \frac{a}{b} \right)\].
Find the tangent of the angle between the lines which have intercepts 3, 4 and 1, 8 on the axes respectively.
Write the coordinates of the image of the point (3, 8) in the line x + 3y − 7 = 0.
The acute angle between the medians drawn from the acute angles of a right angled isosceles triangle is
The angle between the lines 2x − y + 3 = 0 and x + 2y + 3 = 0 is
The equation of the line with slope −3/2 and which is concurrent with the lines 4x + 3y − 7 = 0 and 8x + 5y − 1 = 0 is
The reflection of the point (4, −13) about the line 5x + y + 6 = 0 is
If x + y = k is normal to y2 = 12x, then k is ______.
Point of the curve y2 = 3(x – 2) at which the normal is parallel to the line 2y + 4x + 5 = 0 is ______.
The two lines ax + by = c and a′x + b′y = c′ are perpendicular if ______.
The equation of the line passing through (1, 2) and perpendicular to x + y + 7 = 0 is ______.
The coordinates of the foot of the perpendicular from the point (2, 3) on the line x + y – 11 = 0 are ______.
The intercept cut off by a line from y-axis is twice than that from x-axis, and the line passes through the point (1, 2). The equation of the line is ______.
Find the equation of the line passing through the point (5, 2) and perpendicular to the line joining the points (2, 3) and (3, – 1).
Find the equation of a straight line on which length of perpendicular from the origin is four units and the line makes an angle of 120° with the positive direction of x-axis.
If the equation of the base of an equilateral triangle is x + y = 2 and the vertex is (2, – 1), then find the length of the side of the triangle.
If p is the length of perpendicular from the origin on the line `x/a + y/b` = 1 and a2, p2, b2 are in A.P, then show that a4 + b4 = 0.
The vertex of an equilateral triangle is (2, 3) and the equation of the opposite side is x + y = 2. Then the other two sides are y – 3 = `(2 +- sqrt(3)) (x - 2)`.
Line joining the points (3, – 4) and (– 2, 6) is perpendicular to the line joining the points (–3, 6) and (9, –18).
| Column C1 | Column C2 |
| (a) The coordinates of the points P and Q on the line x + 5y = 13 which are at a distance of 2 units from the line 12x – 5y + 26 = 0 are |
(i) (3, 1), (–7, 11) |
| (b) The coordinates of the point on the line x + y = 4, which are at a unit distance from the line 4x + 3y – 10 = 0 are |
(ii) `(- 1/3, 11/3), (4/3, 7/3)` |
| (c) The coordinates of the point on the line joining A (–2, 5) and B (3, 1) such that AP = PQ = QB are |
(iii) `(1, 12/5), (-3, 16/5)` |
A ray of light coming from the point (1, 2) is reflected at a point A on the x-axis and then passes through the point (5, 3). The co-ordinates of the point A is ______.
