Advertisements
Advertisements
Question
Find the perimeter and area of the quadrilateral ABCD in which AB = 17 cm, AD =9 cm, CD = l2cm, ∠ACB = 90° and AC=l5cm.
Solution
The sides of a quadrilateral ABCD in which AB = 17 cm, AD = 9 cm, CD = 12 cm, ∠ACB = 90° and AC = 15 cm
Here, By using Pythagoras theorem
`BC=sqrt(17^2-15^2) = sqrt(289-225)=sqrt64=8cm`
Now, area of ΔABC=`1/2xx8xx15=60cm^2`
For area of Δle ACD,
Let a = 15 cm, b = 12 cm and c = 9 cm
Therefore, S =`(15+12+9)/2=36/2=18cm`
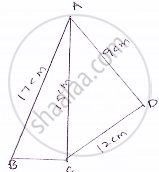
Area of ACD =`sqrt(s(s-a)(s-b)(s-c))`
`=sqrt(18(18-15)(18-12)(18-9))`
`=sqrt(18xx18xx3xx3)`
`=sqrt((18xx3)^2)`
`=54cm^2`
∴ Thus, the area of quadrilateral ABCD = `60 + 54 = 114 cm^2`
APPEARS IN
RELATED QUESTIONS
Radha made a picture of an aeroplane with coloured papers as shown in the given figure. Find the total area of the paper used.
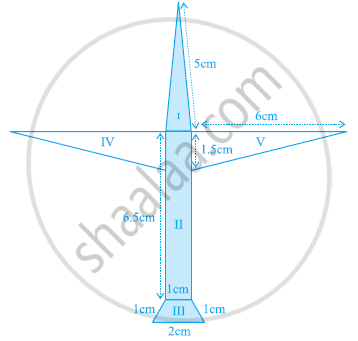
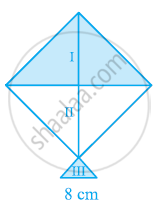
A kite in the shape of a square with a diagonal 32 cm and an isosceles triangles of base 8 cm and sides 6 cm each is to be made of three different shades as shown in the given figure. How much paper of each shade has been used in it?
Find the area of a quadrilateral ABCD is which AB = 3 cm, BC = 4 cm, CD = 4 cm, DA = 5 cm and AC = 5 cm.
Two parallel side of a trapezium are 60 cm and 77 cm and other sides are 25 cm and 26 cm. Find the area of the trapezium.
If each side of a equilateral triangle is tripled then what is the percentage increase in the area of the triangle?
The base of an isosceles right triangle is 30 cm. Its area is
The sides of a triangle are 11 cm, 15 cm and 16 cm. The altitude to the largest side is
A park is in the shape of a quadrilateral. The sides of the park are 15 m, 20 m, 26 m and 17 m and the angle between the first two sides is a right angle. Find the area of the park
The sides of a triangle are 56 cm, 60 cm and 52 cm long. Then the area of the triangle is ______.
The sides of a triangle are 35 cm, 54 cm and 61 cm, respectively. The length of its longest altitude ______.
