Advertisements
Advertisements
Question
Find the area of the region bounded by the ellipse `x^2/4 + y^2/9 = 1.`
Solution
The given ellipse is `x^2/4 + y^2/9 = 1`
Since the given curve is symmetrical about both axes.
∴ Area of ellipse = 4 areas (OABO)
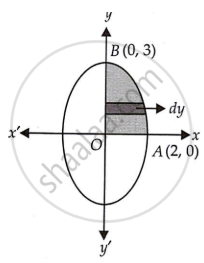
∴ Requied area = 4 Area (OABO) = `4 int_0^3 x dy` ...[by taking horizontal strips]
`4 int_0^3 2/3 sqrt (9 - y^2) dx` `...[x^2/4 + y^2/9 = 1 ⇒ x^2/4 = 1 - y^2/9 ⇒ x = 2/3 sqrt (9 - y^2) (∵ x > 0)]`
`= 4 xx 2/3 [y/2 sqrt (9 - y^2) + 9/2 sin^-1 y/3]_0^3`
`= 4 xx 2/3 [(3/2 (0) + 9/2 sin^-1 (1)) - (0 - 0)]`
`= 4 xx 2/3 [9/2 (pi/2)]`
`= 4 xx (3pi)/2`
= 6π square units
APPEARS IN
RELATED QUESTIONS
Find the area of the region bounded by y2 = 9x, x = 2, x = 4 and the x-axis in the first quadrant.
Find the area of the region bounded by the parabola y = x2 and y = |x| .
Find the area bounded by the curve x2 = 4y and the line x = 4y – 2
Find the area under the given curve and given line:
y = x2, x = 1, x = 2 and x-axis
Find the area of the smaller region bounded by the ellipse `x^2/9 + y^2/4` and the line `x/3 + y/2 = 1`
Find the equation of an ellipse whose latus rectum is 8 and eccentricity is `1/3`
Using integration, find the area of the region {(x, y) : x2 + y2 ≤ 1 ≤ x + y}.
Find the area of the region bounded by the following curves, the X-axis and the given lines: y = `sqrt(16 - x^2)`, x = 0, x = 4
Find the area of the region bounded by the following curves, the X-axis and the given lines:
y = x2 + 1, x = 0, x = 3
Find the area of the region bounded by the parabola y2 = 4x and the line x = 3.
Choose the correct alternative :
Area of the region bounded by y = x4, x = 1, x = 5 and the X-axis is _____.
Fill in the blank :
Area of the region bounded by y = x4, x = 1, x = 5 and the X-axis is _______.
Using definite integration, area of the circle x2 + y2 = 49 is _______.
Fill in the blank :
Area of the region bounded by x2 = 16y, y = 1, y = 4 and the Y-axis, lying in the first quadrant is _______.
State whether the following is True or False :
The area bounded by the curve x = g (y), Y-axis and bounded between the lines y = c and y = d is given by `int_"c"^"d"x*dy = int_(y = "c")^(y = "d") "g"(y)*dy`
Solve the following :
Find the area of the region bounded by the curve y = x2 and the line y = 10.
Solve the following :
Find the area of the region bounded by y = x2, the X-axis and x = 1, x = 4.
Choose the correct alternative:
Area of the region bounded by the curve y = x3, x = 1, x = 4 and the X-axis is ______
Choose the correct alternative:
Area of the region bounded by x = y4, y = 1 and y = 5 and the Y-axis lying in the first quadrant is ______
Choose the correct alternative:
Area of the region bounded by the parabola y2 = 25x and the lines x = 5 is ______
The area of the circle x2 + y2 = 16 is ______
The area of the region bounded by the curve y2 = 4x, the X axis and the lines x = 1 and x = 4 is ______
The area of the region lying in the first quadrant and bounded by the curve y = 4x2, and the lines y = 2 and y = 4 is ______
The area of the region bounded by the curve y2 = x and the Y axis in the first quadrant and lines y = 3 and y = 9 is ______
Find the area of the region bounded by the curve y = `sqrt(2x + 3)`, the X axis and the lines x = 0 and x = 2
Find area of the region bounded by the parabola x2 = 36y, y = 1 and y = 4, and the positive Y-axis
If `int_0^(pi/2) log (cos x) "dx" = - pi/2 log 2,` then `int_0^(pi/2) log (cosec x)`dx = ?
The area enclosed between the curve y = loge(x + e) and the coordinate axes is ______.
The ratio in which the area bounded by the curves y2 = 8x and x2 = 8y is divided by the line x = 2 is ______
`int "e"^x ((sqrt(1 - x^2) * sin^-1 x + 1)/sqrt(1 - x^2))`dx = ________.
Area under the curve `y=sqrt(4x+1)` between x = 0 and x = 2 is ______.
Area of the region bounded by y= x4, x = 1, x = 5 and the X-axis is ______.
If area of the region bounded by y ≥ cot( cot–1|In|e|x|) and x2 + y2 – 6 |x| – 6|y| + 9 ≤ 0, is λπ, then λ is ______.
Area bounded by y = sec2x, x = `π/6`, x = `π/3` and x-axis is ______.
The area (in sq. units) of the region {(x, y) : y2 ≥ 2x and x2 + y2 ≤ 4x, x ≥ 0, y ≥ 0} is ______.
If the area enclosed by y = f(x), X-axis, x = a, x = b and y = g(x), X-axis, x = a, x = b are equal, then f(x) = g(x).
