Advertisements
Advertisements
Question
Find the area of the triangle whose vertices are (-1, 1), (0, 5) and (3, 2), using integration.
Advertisements
Solution
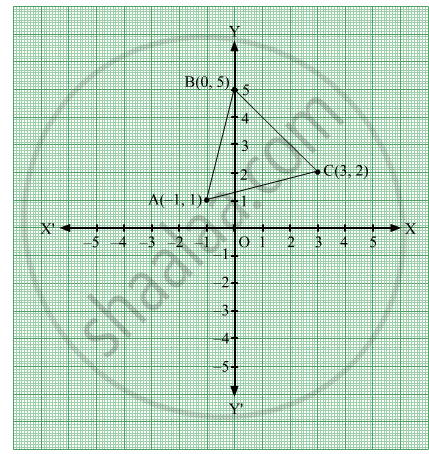
Let A (-1,1), B (0,5) and C (3,2)
The equation of line AB is
y -1 = `(5 -1)/(0+ 1) ("x"+1)`
y = `4"x" + 5`
The equation of line BC is
y - 5 = `(2 -5)/(3 -0) ("x" -0)`
y = `-"x"+5`
The equation of line CA is
y - 2 = `(1 -2)/(-1 -3) ("x" -3)`
y = `("x")/(4) + (5)/(4)`
Required area = Area of ΔABC
The equation of line CA is
y - 2 = `(1 -2)/(-1 -3) ("x" -3)`
y = `("x")/(4) + (5)/(4)`
Required area = Area of ΔABC
= `int_-1^0 (4x + 5) dx + int_0^3 (- x + 5) dx - int_-1^3 ( x/4 + 5/4) dx`
= `[ 2x^2 + 5x ]_-1^0 + [ -x^2/2 + 5x ]_0^3 - [ x^2/8 + 5x/4 ]_-1^3`
= `3 + 21/2 - 39/8 - 9/8`
= `15/2` sq. units
APPEARS IN
RELATED QUESTIONS
Find the integrals of the function:
sin2 (2x + 5)
Find the integrals of the function:
sin 3x cos 4x
Find the integrals of the function:
sin 4x sin 8x
Find the integrals of the function:
`(sin^2 x)/(1 + cos x)`
Find the integrals of the function:
`(cos 2x - cos 2 alpha)/(cos x - cos alpha)`
Find the integrals of the function:
`(cos 2x+ 2sin^2x)/(cos^2 x)`
Find the integrals of the function:
`(cos 2x)/(cos x + sin x)^2`
`int (e^x(1 +x))/cos^2(e^x x) dx` equals ______.
Find `int dx/(x^2 + 4x + 8)`
Evaluate: `int_0^π (x sin x)/(1 + cos^2x) dx`.
Evaluate `int_0^(3/2) |x sin pix|dx`
Differentiate : \[\tan^{- 1} \left( \frac{1 + \cos x}{\sin x} \right)\] with respect to x .
Find `int_ sin ("x" - a)/(sin ("x" + a )) d"x"`
Find: `intsqrt(1 - sin 2x) dx, pi/4 < x < pi/2`
`int "dx"/(sin^2x cos^2x)` is equal to ______.
Evaluate the following:
`int ((1 + cosx))/(x + sinx) "d"x`
Evaluate the following:
`int ("d"x)/(1 + cos x)`
Evaluate the following:
`int (sin^6x + cos^6x)/(sin^2x cos^2x) "d"x`
Evaluate the following:
`int (cosx - cos2x)/(1 - cosx) "d"x`
Evaluate the following:
`int "e"^(tan^-1x) ((1 + x + x^2)/(1 + x^2)) "d"x`
`int (x + sinx)/(1 + cosx) "d"x` is equal to ______.
`int sinx/(3 + 4cos^2x) "d"x` = ______.
`int (cos^2x)/(sin x + cos x)^2 dx` is equal to
Which identity is used for the product of sine and cosine?
What is \(\int \cos^2 x\,dx\)?
What should be done first when evaluating an integral containing a complicated trigonometric expression?
