Advertisements
Advertisements
Question
Find the length of EC.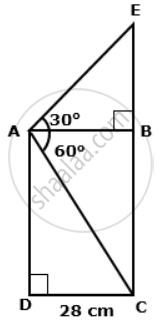
Solution

CD = 28cm
⇒ AB = 28cm
In right ΔABE,
tan30° = `"BE"/"AB"`
⇒ `(1)/sqrt(3) = "BE"/(28)`
⇒ BE = `(28)/sqrt(3)`
In right ΔABC,
tan60° = `"CB"/"AB"`
⇒ `sqrt(3) = "CB"/(28)`
⇒ CB = `28sqrt(3)`
∴ Length of EC
= CB + BE
= `28sqrt(3) + (28)/sqrt(3)`
= `(84 + 28)/sqrt(3)`
= `(112)/sqrt(3)`.
APPEARS IN
RELATED QUESTIONS
If sin 3A = 1 and 0 < A < 90°, find cos 2A
If 3 tan A - 5 cos B = `sqrt3` and B = 90°, find the value of A
If A = B = 60°, verify that: cos(A - B) = cosA cosB + sinA sinB
If A = B = 60°, verify that: sin(A - B) = sinA cosB - cosA sinB
In the given figure, PQ = 6 cm, RQ = x cm and RP = 10 cm, find
a. cosθ
b. sin2θ- cos2θ
c. Use tanθ to find the value of RQ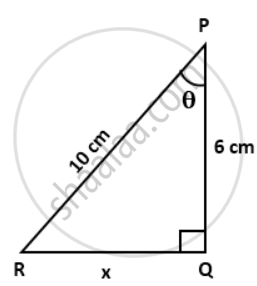
Find the value of: `sqrt((1 - sin^2 60°)/(1 + sin^2 60°)` If 3 tan2θ - 1 = 0, find the value
a. cosθ
b. sinθ
Find x and y, in each of the following figure: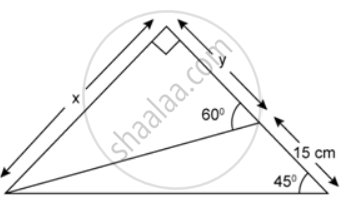
Evaluate the following: sec16° tan28° - cot62° cosec74°
Evaluate the following: `(5sec68°)/("cosec"22°) + (3sin52° sec38°)/(cot51° cot39°)`
Evaluate the following: `(3sin^2 40°)/(4cos^2 50°) - ("cosec"^2 28°)/(4sec^2 62°) + (cos10° cos25° cos45° "cosec"80°)/(2sin15° sin25° sin45° sin65° sec75°)`
