Advertisements
Advertisements
Question
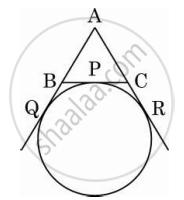
If a circle is touching the side BC of ΔABC at P and is touching AB and AC produced at Q and R respectively (see the figure). Prove that AQ = `1/2` (perimeter of ΔABC).
Solution
Given, circle touching the side BC of ΔABC at point P and AB, AC produced at Q and R, respectively.
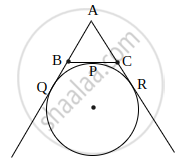
We know, lengths of tangents drawn from on external point to a circle are equal.
∴ AQ = AR ...(i)
BQ = BP ...(ii)
CP = CR ...(iii)
Perimeter of ΔABC = AB + BC + CA
=AB + (BP + PC) + (AR – CR)
= (AB + BP) + PC + (AQ – CP) ...[From equations (i) and (ii)]
= (AB + BQ) + PC + (AQ – CP) ...[From equation (ii)]
= AQ + PC + AQ – PC
= 2AQ
∴ AQ = `1/2` (perimeter of ΔABC)
APPEARS IN
RELATED QUESTIONS
Prove that “The lengths of the two tangent segments to a circle drawn from an external point are equal.”
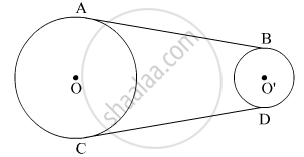
In the figure, AB and CD are common tangents to two circles of unequal radii. Prove that AB = CD.
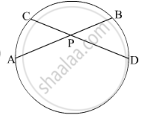
In the given figure PA = 10, PB = 2 and PC = 5. Find PD.
In the figure given below, O is the center of the circle and SP is a tangent. If ∠SRT = 65°, find the value of x, y and Z.

In the given figure, AD is a diameter. O is the centre of the circle. AD is parallel to BC and ∠CBD = 32°.
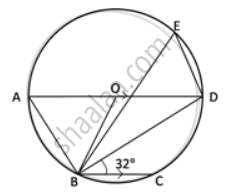
Find: ∠BED
A tangent is drawn from a point at a distance of 17 cm of circle C(0, r) of radius 8 cm. The length of its tangent is ______
A tangent PQ at a point P of a circle of radius 5 cm meets a line through the center O at a point Q such that OQ = 13 cm. Length PQ is ______
In figure, common tangents AB and CD to two circles intersect at E. Prove that AB = CD.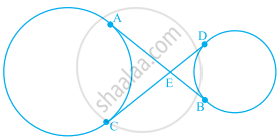
From an external point P, two tangents, PA and PB are drawn to a circle with centre O. At one point E on the circle tangent is drawn which intersects PA and PB at C and D, respectively. If PA = 10 cm, find the the perimeter of the triangle PCD.
Draw two concentric circles of radii 2 cm and 3 cm. From a point on the outer circle, construct a pair of tangents to the inner circle.
