Advertisements
Advertisements
Question
If ∆ABC ~ ∆DEF, AB = 4 cm, DE = 6 cm, EF = 9 cm and FD = 12 cm, find the perimeter of ∆ABC.
Solution
According to the question,
AB = 4 cm,
DE = 6 cm
EF = 9 cm
FD = 12 cm
Also,
∆ABC ∼ ∆DEF
We have,
∴ `("AB")/("ED") = ("BC")/("EF") = ("AC")/("DF")`
⇒ `4/6 = ("BC")/9 = ("AC")/12`
By taking first two terms, we have
⇒ `4/6 = ("BC")/9`
⇒ BC = `((4 xx 9))/6` = 6 cm
And by taking last two terms, we have,
`("BC")/9 = ("AC")/12`
`6/9 = ("AC")/12`
AC = `(6 xx 12)/9` = 8 cm
Now,
Perimeter of ∆ABC
= AB + BC + AC
= 4 + 6 + 8
= 18 cm
Thus, the perimeter of the triangle is 18 cm.
APPEARS IN
RELATED QUESTIONS
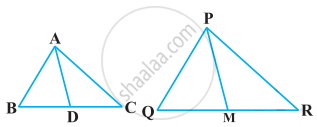
State which pair of triangles in the following figure are similar. Write the similarity criterion used by you for answering the question, and also write the pairs of similar triangles in the symbolic form:
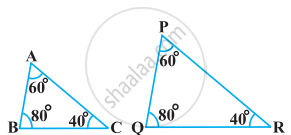
State which pair of triangles in the following figure are similar. Write the similarity criterion used by you for answering the question, and also write the pairs of similar triangles in the symbolic form:
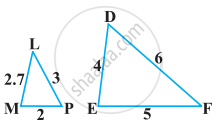
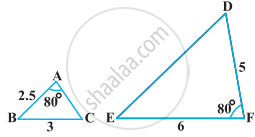
State which pair of triangles in the following figure are similar. Write the similarity criterion used by you for answering the question, and also write the pairs of similar triangles in the symbolic form:
E is a point on the side AD produced of a parallelogram ABCD and BE intersects CD at F. Show that ΔABE ∼ ΔCFB.
In the following figure, ABC and AMP are two right triangles, right-angled at B and M respectively, prove that:

- ΔABC ~ ΔAMP
- `("CA")/("PA") = ("BC")/("MP")`
A vertical pole of a length 6 m casts a shadow 4m long on the ground and at the same time a tower casts a shadow 28 m long. Find the height of the tower.
D is the mid-point of side BC of a ΔABC. AD is bisected at the point E and BE produced cuts AC at the point X. Prove that BE : EX = 3 : 1
In Fig below we have AB || CD || EF. If AB = 6 cm, CD = x cm, EF = 10 cm, BD = 4 cm and DE = y cm, calculate the values of x and y.

If in two triangles DEF and PQR, ∠D = ∠Q and ∠R = ∠E, then which of the following is not true?
Sides AB and BC and median AD of a triangle ABC are respectively proportional to sides PQ and QR and median PM of ΔPQR show that ΔABC ~ ΔPQR.