Advertisements
Advertisements
Question
If cos A = `(2x)/(1 + x^2)`, then find the values of sin A and tan A in terms of x
Solution
cos A = `(2x)/(1 + x^2)`
In the triangle ABC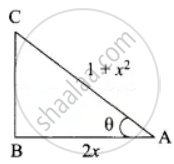
BC2 = AC2 – AB2
= (1 + x2)2 – (2x)2
= 1 + x4 + 2x2 – 4x2
= x4 – 2x2 + 1
= (x2 – 1)2 or (1 – x2)2 ...[using (a – b)2]
BC = `sqrt((x^2 - 1)^2` or `sqrt((1 - x^2)^2`
BC = x2 – 1
The value of sin A = `"BC"/"AC" = (x^2 - 1)/(x^2 + 1)`
tan A = `"BC"/"AB" = (x^2 - 1)/(2x)`
and
BC = 1 – x2
The value of sin A = `(1 - x^2)/(x^2 + 1)`
tan A = `(1 - x^2)/(2x)`
APPEARS IN
RELATED QUESTIONS
If 3cos θ – 4sin = 2cos θ + sin θ Find tan θ.
If A = B = 60°, verify that cos (A − B) = cos A cos B + sin A sin B
If tan θ = `20/21` show that `((1-sin θ + cos θ))/((1+ sin θ +cos θ)) = 3/7`
If 3 cot θ 4 , show that`((1-tan^2theta))/((1+tan^2theta)) = (cos^2theta - sin^2theta)`
If a right ΔABC , right-angled at B, if tan A=1 then verify that 2sin A . cos A = 1
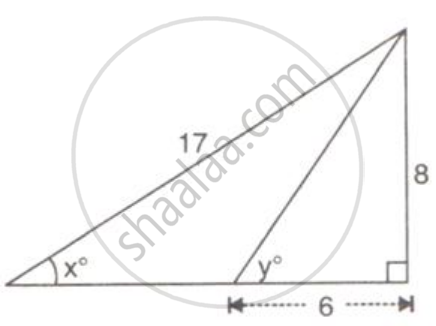
Use the given figure to find :
(i) sin xo
(ii) cos yo
(iii) 3 tan xo - 2 sin yo + 4 cos yo.
In the diagram, given below, triangle ABC is right-angled at B and BD is perpendicular to AC.
Find:
(i) cos ∠DBC
(ii) cot ∠DBA

Using the measurements given in the following figure:
(i) Find the value of sin θ and tan θ.
(ii) Write an expression for AD in terms of θ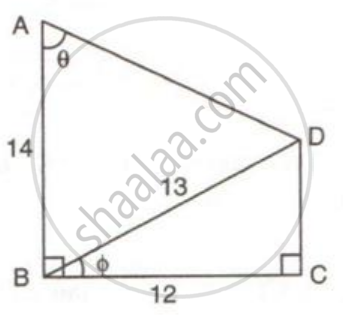
If tan A + cot A = 5;
Find the value of tan2 A + cot2 A.
If sin A = `(7)/(25)`, find the value of : `(2"tanA")/"cot A - sin A"`
